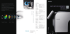Growth increments in Gomphotherium tusks and implications for late

Transcription

Palaeogeography, Palaeoclimatology, Palaeoecology 156 (2000) 327–348
www.elsevier.nl/locate/palaeo
Growth increments in Gomphotherium tusks and implications
for late Miocene climate change in North America
David L. Fox *,1
University of Michigan, Department of Geological Sciences and Museum of Paleontology, Ann Arbor, MI 48109, USA
Received 4 November 1998; received in revised form 27 September 1999; accepted for publication 28 September 1999
Abstract
Changes in mammalian faunas in North America during the late Miocene are thought to have been caused by the
replacement of woodland habitats with grassland or steppe. The proposed cause of this habitat shift is the transition
from the relatively low-seasonality early Miocene climate to a more highly seasonal climate regime by the end of the
Miocene. Tusks, which are highly modified incisor teeth, of late Miocene Gomphotherium (Mammalia, Proboscidea)
were sectioned to document the nature of tusk growth in this genus and to test for patterns of seasonal growth. Both
the dentin and enamel of Gomphotherium tusks preserve incremental growth lines. Dentin has increments on three
scales, inferred to represent annual (first-order), weekly (second-order), and daily (third-order) periodicities. Luminance
and second-order increment thickness profiles were measured on transverse thin sections of tusk dentin viewed at low
magnification, and the data were examined with bivariate plots and autocorrelation. Autocorrelograms of luminance
data support the consistent identification and measurement of second-order increments within and among the tusks
sampled. Profiles of second-order increment thickness in three tusks from the Barstovian North America Land
Mammal Age (NALMA) show no indication of seasonal patterns of growth. One tusk from the late Clarendonian
and one from the early Hemphillian NALMA have patterns of growth consistent with an increase in seasonality
during the late Miocene, an interpretation supported by autocorrelation of the increment thickness data from these
tusks. The growth patterns are consistent with the hypothesized changes in North American climate during the late
Miocene and are suggestive of an increase in aridity and development of a wet season. Tests of this hypothesis are
suggested. © 2000 Elsevier Science B.V. All rights reserved.
Keywords: Miocene; North America; paleoclimate; proboscidea; seasonality
1. Introduction
The middle to late Miocene, from about 15 to
5 Ma, was a time of significant faunal change
among North American mammals. Generic diversity declined from the Cenozoic high of approxi* Fax: +1-831-459-3074.
E-mail address: [email protected] (D.L. Fox)
1 Present address: University of California, Santa Cruz,
Department of Earth Sciences, Santa Cruz, CA 95064, USA.
mately 140 genera (Stucky, 1990) during the
Barstovian North American Land Mammal Age
(NALMA; 14.5–11.5 Ma) in a series of extinctions
that began in the Clarendonian NALMA (11.5–
9 Ma) and culminated in the extinctions at the end
of the late Hemphillian NALMA (9–4 Ma)
( Tedford et al., 1987). This late Hemphillian
extinction, which affected as many as 65 genera,
was more devastating than the better known
Rancholabrean event at the close of the last glacial
period ( Webb, 1984a; Alroy, 1992). Extinction of
0031-0182/00/$ - see front matter © 2000 Elsevier Science B.V. All rights reserved.
PII: S0 0 3 1 -0 1 8 2 ( 9 9 ) 0 0 14 8 - 0
328
D.L. Fox / Palaeogeography, Palaeoclimatology, Palaeoecology 156 (2000) 327–348
large herbivores was dramatic. Many species with
brachydont ( low-crowned) dentition typical of
browsers, such as leptomerycid artiodactyls, disappeared early during the late Miocene, and almost
all browsers were extinct by the end of the
Clarendonian. Most lineages that survived, such
as equids, had hypsodont (high-crowned ) teeth
typical of grazers and locomotor adaptations for
cursoriality. Thus, many of the herbivores that
persisted into the Pliocene were well adapted for
fibrous or gritty diets and open habitats ( Webb,
1984b). The patterns of survivorship have been
inferred to result from the replacement of woodland savanna in the mid-continent with pure grassland or steppe habitats due to some combination
of cooling, drying and an increase in the seasonality of temperature and/or precipitation during the
late Miocene ( Webb, 1977; Janis, 1989).
The changes among North American mammals
during the Miocene are broadly correlative with
records, both marine and terrestrial, indicating
climate, habitat, and faunal changes globally. The
oxygen isotope composition of benthic foraminifera dropped sharply during the middle Miocene
(Miller et al., 1987; Wright et al., 1992), marking
a transition from warmer climates earlier in the
Cenozoic to the cold climates of the PlioPleistocene. Sea levels fluctuated widely and then
fell over this same interval ( Haq et al., 1987). The
East Antarctic Ice Sheet also experienced a period
of fluctuation in size followed by expansion during
the middle Miocene, possibly in response to
changes in deep water circulation ( Woodruff and
Savin, 1989; Flower and Kennett, 1994). These
changes in the marine record are associated with
increased turnover among planktonic foraminifera
( Wei and Kennett, 1986), and mammalian faunas
from continents other than North America, particularly the well-documented faunas of Pakistan,
also exhibit significant turnover during the same
interval (Barry et al., 1985). Terrestrial floras from
around the world indicate the geographic expansion of xeric, or dry habitat, plants during the
middle and late Miocene ( Wolfe, 1985; Singh,
1988). Paralleling the paleobotanical evidence, the
carbon isotope composition of paleosol carbonates
and the teeth of mammalian herbivores on many,
but not all, continents record marked increases in
the abundance of tropical C grasses beginning
4
around 7 Ma (Cerling et al., 1997), consistent with
the growth of continental grasslands during the
late Miocene. While all of these records, including
the succession of mammalian faunas in North
America, certainly reflect climate change on some
spatial and temporal scales, none records directly
and unambiguously the changes in annual climatic
variability postulated to have contributed to the
biotic responses in the late Miocene.
In this paper, I discuss a source of evidence
that may be sensitive to seasonal variability in
climate and hence may shed light on the nature of
climate change during the Miocene: incremental
growth features in the dentin of the upper tusks
of the proboscidean Gomphotherium. Proboscidean
tusks are highly modified incisor teeth that are
composed primarily of the tissue dentin. The
growth and structure of tusks have been studied
in a number of proboscideans, including mastodons (Mammut americanum) and mammoths
(genus Mammuthus) of the late Pleistocene of
North America, as well as both extant species of
elephant (Elephas maximus and Loxodonta africana) (Fisher, 1984, 1987, 1988; Koch, 1989; Fox
and Fisher, 1994). The tusks of many late
Pleistocene mastodons and mammoths have patterns of growth increments in tusk dentin that are
consistent with seasonal changes in the rate of
dentin apposition, presumably reflecting seasonality of climate and food-resource abundance in
parts of North America during the late Pleistocene
( Fisher, 1987, 1990, 1996; Koch et al., 1989). As
discussed below, similar phenomena have been
documented in the teeth of a variety of mammals.
Using such growth patterns as an analog, it is
possible that gomphothere tusks may similarly
record the influence of climate and habitat conditions on growth.
This study has two goals. The first is to describe
the internal structure of Gomphotherium tusks. The
tusk dentin of proboscidean taxa that have been
studied are composed of incremental laminae organized on three spatial scales. While gomphothere
tusks are somewhat different from others in having
a continuous band of enamel along the lateral
surface, their general form suggests that gomphothere tusks should be structurally similar to those
of other proboscideans. The second, more significant goal is to use the pattern of variation in the
thickness of subannual growth increments to infer
growth rate. Analysis of a suite of gomphothere
tusks from localities spanning the middle to late
Miocene provides a means to detect changes in
the degree of seasonal patterns of tusk growth,
which provides a means of testing the hypothesized
trend toward increasing annual variation in
climate.
2. Growth increments in mammalian teeth
The growth of the mineralized dental tissues of
mammals — cementum, dentin and enamel — is
an accretionary process that varies in duration
from weeks to whole lifetimes among different
taxa. At a gross scale, mineralization is broadly
similar in all three tissues; hydroxyapatite crystals
nucleate and grow in an organic matrix deposited
on the growing surface of the tooth by secretory
cells. Regular disturbances to or variations in
physiology from both external and internal sources
during deposition and mineralization of these tissues are often expressed as incremental growth
banding. Incremental features appear as alternating light and dark zones in the mineralized tissue,
but the contrast relationship (dark–light or light–
dark) is dependent on the type of illumination
(transmitted, reflected, with/without crossed
Nichols), and, in the case of fossil teeth, the quality
of preservation. Although growth lines in teeth
have been known since the nineteenth century
work of Owen (1845) and others, temporal periodicities of these features were not recognized until
later (Gysi, 1931; Kimura, 1939; Schour and
Hoffman, 1939; Okada, 1943; Sheffer, 1950; Laws,
1952). To date, temporally periodic increments
have been identified in at least 89 species of marine
and terrestrial mammals ( Fox, unpub. review). In
most species, only annual periodicities have been
identified, possibly stemming from the common
use of incremental features by mammalogists for
aging individuals (e.g. Klevezal and Kleinenberg,
1969; Perrin and Myrick, 1980). However, subannual increments with lunar monthly, fortnightly
and daily periodicities, in some cases hierarchically
329
arranged, are known from several extant species
(Laws, 1962; Sheffer, 1970; Koch, 1989; Klevezal
and Mina, 1990; Fox and Fisher, 1994).
Neither the structural basis of color banding in
teeth nor the biological basis for its periodic
expression is well understood. No consensus currently exists on either issue for any dental tissue.
Lieberman (1994) has reviewed hypotheses for
annual increments in cementum and, based on
controlled experiments, proposed that incremental
features in cementum result from changes in occlusal stresses associated with diets that vary seasonally in nutritional quality and physical properties.
This explanation cannot apply to dentin and
enamel, both of which have incremental features
that are produced before tooth eruption or, in the
case of proboscidean tusks, in the absence of
occlusal stress. The generally accepted explanation
for annual increments in dentin is that they reflect
seasonal variation in the density of the mineral
portion of the tissue as a result of seasonal variation in nutritional stress and growth rate of an
individual (Laws, 1962; Klevezal and Kleinenberg,
1969). This idea dovetails the structural origin of
annual increments with the biological basis of the
periodicity. Explanations of the structural basis of
subannual increments in dentin and enamel include
physico-chemical factors inherent to the process
of crystallite growth (Mummery, 1924), variations
in the degree of mineralization, variations in the
orientation of collagen fibers in the organic matrix
(Schmidt and Kiel, 1971) and variations in the
chemical composition of the mineral itself (Boyde,
1979). Explanations for subannual periodicities
include a circadian rhythm of body-fluid alkalinity
(Okada, 1943), the daily timing of feeding (Miani
and Miani, 1972; Klevezal, 1980), and periodicities
in the body-fluid concentration of hormones possibly related to the growth of mineralized tissues
( Rosenberg and Simmons, 1980; Halberg, 1983).
Interactions of different physiological systems with
circadian, circaseptan (‘about seven’ day) and
other rhythms could result in features with the
various periodicities that have been observed in
teeth, but the identity and nature of the physiological systems remain elusive.
Despite uncertainties associated with both the
physical and biological genesis of incremental fea-
330
tures, they can still be used as sources of data on
the lives of animals, provided that the temporal
signal is consistently recognized. Based on the
recognition of annual increments, mammalogists
frequently use growth bands as a means of assessing the age structure and dynamics of wild populations in a variety of mammals, most commonly
marine species (e.g. Hewer, 1964; Marsh, 1980;
Slooten, 1991). Archaeologists use annual
increments in teeth from archaeological sites to
examine temporal patterns of site and resource
utilization in past human populations (e.g. Saxon
and Higham, 1969; Fisher, 1987; Lieberman, 1993;
Burke and Castanet, 1995), and physical anthropologists use them to examine tooth growth and
age at death of fossil hominids (e.g. Dean, 1987).
Incremental features have been examined in teeth
of some fossil and subfossil mammals, such as the
beaver (Mayhew, 1978), but the most detailed
work to date is on the tusks of late Pleistocene
proboscideans.
3. Growth increments in proboscidean tusks
Micro- and macroscopic analyses of the tusks
of mastodons, mammoths, and both living species
of elephant have identified a hierarchical system
of growth increments in the dentin ( Fisher, 1984,
1987, 1988, 1990, 1996; Koch, 1989; Fox and
Fisher, 1994). Growth banding occurs in the tusks
of these animals on three spatial scales and is
visible as alternating dark–light couplets, as is the
case in the teeth of many other mammal teeth.
The growth bands parallel the pulp cavity and
imbricate along the length of the tusks so that the
earliest deposited — hence oldest — dentin is
distal, and the dentin becomes younger towards
the surface of apposition in the pulp cavity.
Borrowing the conventions of sequence stratigraphy, the largest and most inclusive increments are
referred to as first-order increments. The thickness
of these increments is on the order of several
millimeters in cross-section. At the next tier in the
hierarchy, second-order increments are on the scale
of tenths of millimeters in thickness. Third-order
increments are the smallest and are tens of microns
in thickness.
The hierarchy of increments is not only spatially, but also temporally, periodic. The full arguments have been presented elsewhere (Fisher, 1987;
Koch, 1989; Koch et al., 1989) but will be briefly
reviewed here. The strongest evidence is the consistent numerical relation between the three scales.
First-order increments are composed of either 52,
26 or 13 second-order features and about 364
third-order increments. Variability in the number
of second-order increments is dependent on individual and species variation and whether a sample
is from molar or tusk dentin. In cases of 52 secondorder increments per first-order feature, each
second-order increment is composed of about
seven third-order increments; in cases of 26 secondorder increments per first-order increment, each
second-order increment is composed of about 14
third-order increments; and in cases of 13 secondorder increments per first-order increment, each
second-order increment is composed of about 28
third-order increments. These numbers indicate
that first-order increments are annual features,
second-order increments are weekly, fortnightly or
monthly and third-order increments are daily. As
discussed above, analogous features and periodicities are known in a variety of other mammals.
This temporal interpretation of growth
increments is corroborated by two lines of evidence. The first is the pattern of variation in the
oxygen isotope composition of tusk dentin. The
d18O values of samples removed following growth
lines have a cyclic pattern in which the lowest
values correspond to winter growth and the highest
values correspond to summer, the season of growth
being inferred from the color and thickness of the
first-order increments (e.g. Koch et al., 1989,
Fig. 3) and by analogy with extant mammals. The
correlation between the variation in d18O and the
color pattern of first-order increments strongly
supports the interpretation of first-order
increments as annual features. The second line of
evidence is the pattern of variation in second-order
increment thickness, and its correlation to both
the pattern of oxygen isotope variation and the
color banding of first-order increments. Plots of
increment thickness versus sequential increment
number (increment thickness profiles) show a characteristic rise and fall over periods of about 26
increments in individuals interpreted to have fortnightly increments (e.g. Koch et al., 1989, Fig. 2).
Sets of narrow increments correlate with the
inferred winter portions of first-order increments
and are presumed to indicate slow growth that
results from winter nutritional stress; the broader
high growth rate periods correlate to the spring–
autumn portions of the first-order increments and
may result from the greater availability of food
during the growing season. Thus, the cyclic patterns of oxygen isotope composition and increment
thickness represent intra-annual or seasonal variation in body-water isotope composition and
growth rate and, and corroborate the temporal
interpretation of both first- and second-order
increments.
4. Implications for Gomphotherium tusks
The recognition of temporally periodic growth
increments and seasonal growth patterns in the
tusks of late Pleistocene proboscideans suggests
that tusks of Miocene proboscideans, such as
Gomphotherium, may be useful as indicators of the
hypothesized climate change during the late
Miocene. It must first be established that
Gomphotherium tusks grew in a manner like those
of later proboscideans and that the tusks have
growth increments that are spatially periodic. Plots
of subannual increment thickness could then be
used to examine temporal periodicity and changes
in tusk growth rate through time. The hypothesis
of increasing seasonality during the late Miocene
predicts a straightforward change in the pattern
of gomphothere tusk growth, assuming a response
to seasonality similar to that documented in the
tusks of late Pleistocene mastodons and mammoths ( Fisher, 1987, 1990, 1996; Koch et al.,
1989). Barstovian specimens should show either
low-amplitude cyclicity or irregular variability with
no discernible pattern in subannual increment
thickness. Increasing seasonality during the
Clarendonian and Hemphillian land mammal ages
would be evident as increment thickness profiles
with recurring, paired intervals of high and low
growth with an annual periodicity inferred from
the number of included subannual increments.
331
Behavioral factors, such as migration, seasonal
dietary shifts, and musth and other aspects of
reproductive behavior, could weaken or even mask
patterns of seasonal growth.
5. Materials and methods
5.1. Specimens
Seven upper tusks of the genus Gomphotherium
from localities in Nebraska, Oklahoma and Texas
were analyzed in this study. Unlike late Pleistocene
and Recent proboscideans, gomphotheres had permanent upper and lower tusks throughout life.
Only upper tusks were used because they are
generally larger than the mandibular tusks, and
increments might therefore be more conspicuous
and easier to analyze. Also, only the upper tusks
have a lateral enamel band, allowing for investigation of both enamel and dentin in each individual.
The specimen numbers, localities and ages for the
seven tusks are given in Fig. 1. Due to the currently
confused and inexact state of gomphothere taxonomy, attribution of these specimens to species is
Fig. 1. Specimen numbers and biostratigraphic position of the
localities arranged by North American Land Mammal Ages
(NALMA) with absolute ages indicated in millions of years
(Ma). The order of specimens within subdivisions of the landmammal ages is arbitrary and should not be taken as indicative
of superpositional relationships among the localities. Age
assignments are based on Corner (1976) and Tedford et al.
(1987); the radiometric scale is based on Woodburne (1987).
332
not currently possible. Six of the seven specimens
have been previously assigned only to the subfamily Gomphotheriinae. One, UNSM 88511, was
attributed to G. osborni by Corner (1976).
However, according to Tobien (1972), all named
species of Gomphotherium in North America can
be accommodated within G. producutus. Although
this issue awaits a rigorous analysis, the aforementioned similarities in the tusks of elephantids and
mammutids suggest that analysis of closely related
congeneric species is not a major concern.
Specimens were selected based on quality of
preservation, although judgment of condition from
external appearance is difficult. Badly cracked or
weathered tusks were rejected, as were extremely
heavy specimens that had probably undergone
substantial post-depositional alteration and permineralization. An additional criterion for inclusion was suitability for shipment to the University
of Michigan, leading to exclusion of large tusks
from old individuals.
5.2. Sectioning
In order to examine the internal structure and
pattern of growth of the enamel and dentin, it was
necessary to section tusks and prepare thin sections. Tusks were embedded in plaster and then
sectioned longitudinally on a rock slabbing saw
using a non-aqueous cutting lubricant. Plaster was
used because it provides adequate support for
potentially friable specimens, yet is easily removed
later. A non-aqueous lubricant was used because
of the potential for water absorption to cause
expansion and damage dry fossil material (Fisher,
1988). The longitudinal cut was oriented to bisect
the enamel band perpendicularly and to pass just
to one side of the tusk axis so that one half of the
tusk actually contained the axis. Sample blocks
for thin sections were removed by making a pair
of transverse cuts (i.e. perpendicular to the tusk
axis) approximately 1.5 cm deep in the tusk section
containing the axis. These cuts were then joined
by a single longitudinal cut to liberate a sample
block. Thin sections on glass slides were prepared
from individual sample blocks following standard
thin sectioning techniques. The finished thickness
of slides was not standardized. Rather, slides were
thinned to achieve the optimal expression of
second-order increments, generally occurring at a
thickness of about 0.3 mm. Thinning beyond this
weakened or eliminated the second-order features,
though it did enhance third-order increments.
However, these smallest scale increments are variably preserved, and second-order increments are
preferred for evaluation of seasonal patterns of
growth. Because the scale of first-order increments
is larger than the field of view under even low
magnification, optimizing their expression was not
a concern in thinning.
5.3. Increment thickness
As discussed below, gomphothere tusks were
found to have three scales of laminar features,
much like Pleistocene and Recent tusks. The
pattern of variation, or lack of pattern, in secondorder increment thickness was used to assess the
degree of seasonal growth in Gomphotherium tusks.
This approach is based on the utility of secondorder increment thickness in Mammut and
Mammuthus for identifying seasonal growth. All
analyses of thin sections were carried out at a
magnification of 25×. Second-order increment
thickness was measured on transverse thin sections
using digitized video images and Optimas image
analysis software ( Vers. 4.1) from Optimas Corp.
In this procedure, an image of a thin section in
transmitted light was captured by a video camera
mounted directly on a petrographic microscope
and the contrast and brightness of the image
adjusted with Optimas image-processing controls
to maximize the contrast between light and dark
portions of second-order increments. Luminance
was then measured by Optimas along a transect
perpendicular to the growth banding at 0.0071 mm
intervals. Markers were placed along the transect
at the midpoint of the dark parts of each
increment, which are generally more discrete than
the accompanying light portion ( Fig. 2A). The
proper position for markers is judged both by eye
from the video and microscope images and from
the luminance profile measured by Optimas, which
can be viewed while evaluating the transect
( Fig. 2B). Because of local changes in the preservation and prominence of growth lines, luminance
values along parts of the transect can be uninformative or even misleading. However, by following
faint or ambiguous lines across a thin section, it is
possible to mark increments accurately even when
the luminance profile is not helpful. Optimas
records the position of each marker along the
transect and exports both luminance and increment
marker data to a spreadsheet. The data from each
slide were collected in a series of discrete, but
overlapping, transects which were then pieced
together into a continuous record. This continuous
record consists of the distance, in millimeters, of
each increment marker from the beginning of the
first discrete transect. Increment thickness is calculated by subtracting the distance along the continuous transect of successive pairs of increment
markers. These values are then plotted against
increment number with the earliest-formed
increment at the origin to generate an increment
thickness profile ( Fig. 2C ).
5.4. Autocorrelation
Autocorrelation was used both to evaluate the
placement of second-order increment markers on
luminance transects from individual screens and
to examine the second-order thickness data for
periodicities consistent with seasonal growth.
Autocorrelation is a simple time series analysis in
which a time series is correlated with itself after a
displacement in the time dimension. The autocorrelation coefficient is calculated between pairs of
values y and y , where i is the position in the
i
i+t
time series and t, or the lag, is the number of
observations by which the time series is offset from
itself. The equation for the autocorrelation coefficient at a given lag, r , is
t
∑ ( y y )∑ y ∑ y
i i+t
i
i+t
r=
t E[∑ y2 −(∑ y )2][∑ y2 −∑ y )2]
i
i
i+t
i+t
(Davis, 1986).
Autocorrelation coefficients at successive lags
are conventionally plotted on an autocorrelogram,
which has r on the ordinate and t on the abscissa
t
(Fig. 2D). As with a standard correlation coefficient, r ranges from 1.0 to −1.0. The value of r
t
t
is necessarily 1.0 at t=0, and it decreases with
333
increasing lag. For random or aperiodic data, r
t
will have an arbitrarily small value at t=1 and
will vary randomly about 0 at increasing lag. Any
smoothness in the data will result in a more
gradual decline in r . Peaks (or valleys) in r after
t
t
a short decline due to smoothness may indicate
cyclicity in the data at a wavelength corresponding
to the lag. A standard Z statistic can be calculated
to assess the statistical significance of the autocorrelation coefficient at a given lag, and hence can
be used to distinguish periodic structure in a data
set from random variation in r . The equation for
t
the Z statistic at a given lag t is
Z =r En−t+3
t t
(Swan and Sandilands, 1995) where r is the autot
correlation coefficient at that lag, and n is the
number of observations in the data set. The critical
values for Z at a=0.05 are ±1.96. All autocorrelt
ograms in this paper have both the Z statistic and
the critical values plotted in addition to r . Any
t
lag with a Z statistic that crosses the critical values
is statistically consistent with a periodicity in the
data at that lag. For the luminance data, r should
t
be high and Z statistically significant for lags that
t
correspond to the mean increment thickness measured for a transect. For example, in the case of
the sample data in Fig. 2, the mean second-order
increment thickness is 0.076 mm. Given that luminance is measured along the transect in 0.0071 mm
intervals, the mean increment thickness implies
that lags 10 and 11 should have a high r and
t
significant Z . The peak in r and Z over lags 10–
t
t
t
14 corresponds to expectation, confirming the
impression from Fig. 2A and B that the variation
in luminance is regular, and supports the identification of second-order increments. For the
increment thickness data, the expectation is that
specimens that have observable first-order or
annual increments and that have a regular variation in the size of second-order increments should
have a high r and significant Z at lags correspondt
t
ing approximately to a year, either 13, 26 or 52,
depending on the temporal value of the secondorder increments.
Autocorrelation coefficients were calculated for
only a subset of the individual luminance profiles
334
as many of the individual screens of data cross
areas with either cracks or unusual luminance or
contrast due to diagenesis. Such transects result in
aberrant, indistinct, or inconsistent luminance profiles that would result in spurious or meaningless
autocorrelograms. A total of seven luminance transects from three individuals were judged suitable
for autocorrelation. The second-order increment
thickness profiles from all seven tusks were analyzed with autocorrelation.
Three restrictions apply to autocorrelation.
First, autocorrelation should only be used on data
sets with at least 50 observations, and the lag
should not exceed one-quarter the length of a data
set (Davis, 1986). This restriction is easily met as
the shortest luminance transect examined with
autocorrelation had 247 luminance measurements,
and the shortest incremental thickness profile had
175 measurements. Second, any linear trend in the
data should be removed prior to analysis, as such
a trend will cause a steady decline in r with
t
increasing lag and hence will mask any pattern in
the data (Swan and Sandilands, 1995). Linear
trends can be introduced into the luminance data
by uneven illumination and by local variability in
the preservation of the sample; linear trends can
be introduced into the increment thickness data
by long-term trends in the growth of an individual
that are distinct from and overlay sub-annual
patterns. Finally, a more important restriction is
that the spacing of observations be constant
(Davis, 1986). For the luminance data, this is
unproblematic due to the constant sampling
interval (0.0071 mm) used in collecting the data
with Optimas; a constant interval in the spatial
domain substitutes for a constant interval in the
temporal domain without violating the restriction
of application to a constant interval. For the
increment thickness data, the assumption is that
all features marked are second-order increments
335
and hence represent the same temporal spacing.
All autocorrelation coefficients and detrending
(when necessary) were calculated using SYSTAT
( Wilkinson, 1990).
6. Results
6.1. Geometry of growth
Longitudinally sectioned tusks, as well as analysis of thin sections, indicate that the basic mode
and geometry of growth of the tusks of
Gomphotherium are essentially the same as in other
proboscideans (e.g. Fisher, 1987; Koch, 1989),
with the exception of the continuous lateral enamel
band. Fig. 3A is an idealized representation of a
sectioned gomphothere tusk indicating the
arrangement of the mineralized tissues. The tusk
is mostly composed of dentin, which is deposited
at the surface of the conical pulp cavity. Two
specimens ( F:AM 129672 and 129673) preserve
open pulp cavities, indicating that the tusks continued growing well into adulthood and, based on
comparison with Pleistocene and Recent tusks,
implying they were evergrowing. Fig. 3B illustrates
the open pulp cavity of F:AM 129672, in which a
plug of loose, coarse sand is visible. Growth
increments in the dentin parallel the pulp cavity,
as suggested by the cracks that follow the growth
banding in Fig. 3B. Such cracking is common in
fossil tusks and is due to differential drying and
shrinking of the interior and exterior of the tusk
(D.C. Fisher, pers. commun., 1999). Enamel is
deposited along the lateral surface of the dentin in
a band several centimeters wide, 1–3 mm thick,
and continuous along the length of the tusk. The
geometry of the surface of enamel apposition is
inferred from the increments in the enamel, as
discussed below. Several specimens preserve a thin
Fig. 2. Example of a screen of data and its results. (A) Digitized image of a portion of F:AM 129670 viewed in transmitted light at
25× as it appears on the video monitor. Growth increments run vertically and the enamel dentin junction is to the right. The transect
is 1.85 mm long and 24 increments are marked with Vs at the dark line of each increment. Increments thus span the distance between
successive V markers. (B) Luminance profile for the transect in (A). (C ) Sequential increment thickness for the increments marked
in (A), beginning with the increment furthest to the left. The average increment thickness is 0.076 mm. (D) Autocorrelogram with
plot of Z statistic and critical values of Z for the luminance data plotted in (B). The peak in r and Z for t=10–14 corresponds to
t
t
t
an implied increment thickness of 0.071–0.099 mm, which includes the measured mean increment thickness for the transect in (A).
336
Fig. 3. (A) Schematic diagram of the proximal end of a gomphothere tusk illustrating the basic geometry of tusk growth. Only firstorder or annual increments are shown. The angle of increments in enamel, thickness of the enamel and cementum, and the degree
of contrast and scale of the first-order increments are exaggerated for clarity. (B) Longitudinally cut surface of F:AM 25729. The
distal end of the tusk is to the right, and the pulp cavity extends the length of this section of tusk. The figured portion of the tusk
is approximately 30 cm in length.
coating of cementum over the dentin proximally,
but this is lost distally by abrasion in life; it is not
clear if the cementum also overlapped the enamel.
6.2. Increments
Growth banding is evident in both enamel and
dentin. In both tissues and at all scales, the
increments appear as alternating zones of light and
dark mineral. Fig. 4A and B are photomicrographs
(transverse and longitudinal, respectively) of
enamel on the same sample block from F:AM
129673. The increments in enamel are much
smaller and less regular than those in dentin. The
increments are approximately planar features that
parallel the irregular enamel–dentin junction in
transverse section, but intersect it at an acute angle
(~7°) proximally in longitudinal section. This lowangle proximal inclination of the increments relative to the enamel–dentin junction (Fig. 4B) is the
basis for the orientation of the surface of enamel
apposition in Fig. 3A. Evident in both photomicrographs of enamel are the faint traces of the enamel
prisms, which run in curving paths from the
enamel–dentin junction toward the exterior surface
of the enamel at the top. In parts of both photo-
337
Fig. 4. Photomicrographs of the enamel of F:AM 129671. Magnification is 40×. (A) Transverse view with the undulatory enamel–
dentin junction at the bottom. (B) Longitudinal view with the proximal portion of tusk to the left. Note the low inclination of the
increments in the proximal direction. The scale bars are 0.5 mm.
micrographs, there is the suggestion of two scales
of incremental features in enamel. Due to their
smaller scale and more irregular expression, the
increments in enamel were not analyzed for seasonal patterns of growth. However, recognition of
the pattern of growth and scale of lamination in
the enamel, and its relation to the more regular
incremental features in dentin, is important for
planned studies of the oxygen and carbon isotope
composition of gomphothere tusks.
Increments occur in the dentin at three spatial
scales and are arranged hierarchically ( Fig. 5A–
C ). Only two specimens have portions that clearly
exhibit first-order increments: F:AM 129671
(Clarendonian) and F:AM 129673 (Hemphillian).
The inconsistent expression of first-order features
could be a result of diagenetic alteration of the
dentin. Alternatively, the degree of seasonality
may have varied from year to year so that the
environmental signal leading to first-order features
may not have been as strong or regular from year
to year. The appearance and scale of the firstorder increments are similar to those in other
proboscideans, as shown by the transverse view of
a sample from F:AM 129673 in Fig. 5A. Each
increment is visible in transmitted light as a narrow
dark zone and a broader light zone. Measured
under a microscope with dial calipers, the thickness
of the increments labeled ‘a’ and ‘b’ in Fig. 5A are
3.7 and 4.2 mm, respectively. The single first-order
increment visible in F:AM 129671 (not figured )
measures 4.0 mm. These measurements, though
few, are within the range of variation for firstorder increments in Mammut and Mammuthus (3–
8 mm; D.C. Fisher, pers. commun., 1999) and
suggest that they might represent annual periodicities in dentinal deposition.
A notable non-incremental feature in Fig. 5A is
the checkerboard pattern of the Schreger bands
that results from the undulation of the dentinal
tubules and which is characteristic of proboscidean
ivory. The only specimens in which the Schreger
banding is not visible are UNSM 88511 and F:AM
99059. Both of these Barstovian specimens are
altered by silicification. The loss of Schreger banding is somewhat puzzling, as the other Barstovian
specimen, F:AM 129670, has also been heavily
altered but still expresses the Schreger banding. A
possible explanation is differential diagenetic infilling of the dentinal tubules, which would obscure
the checkerboard pattern of the Schreger bands.
At low magnification, the second-order
increments within the first-order increments look
like tree rings or the grooves in a vinyl record
( Fig. 5A). At higher magnification ( Figs. 2A and
5B), the character and regularity of these features
are clear. All specimens examined in this study
had clearly recognizable second-order increments.
338
Fig. 5. Examples of growth increments in Gomphotherium tusks. Specimens chosen for clarity at each scale. (A) Annual banding in
a transverse surface of F:AM 129673 viewed in transmitted light. The block is from the proximal end of the tusk. The lateral enamel
band is at the top and is translucent in transmitted light. The curved surface at bottom is the surface of the pulp cavity. Two complete
first-order increments are labeled ‘a’ and ‘b’. The dark zone and part of the light zone of a third first-order increment are evident
above ‘a’, and the light zone of a fourth is evident below ‘b’ . Cracks in the dentin are evident towards the pulp cavity and below
the enamel–dentin junction. Second-order increments are visible across most of this block as roughly horizontal dark and light lines.
The checkerboard pattern is Schreger banding. The scale bar is 5.0 mm. (B) Photomicrograph of a thin section of F:AM 129670 in
transverse view at 40× magnification. The prominent light and dark bands running horizontally are second-order increments (18
are visible). The faint vertical lineation is caused by dentinal tubules, which run from the axial portion of the section at bottom
towards the enamel–dentin junction toward the top. Third-order increments are visible within a number of the second-order increments.
The scale bar is 0.5 mm. (C ) Photomicrograph of a thin section of F:AM 129671 in transverse view at 100× magnification. Growth
increments run horizontally. The broad dark and light bands are second-order increments (seven are visible) and the more numerous,
narrow couplets are third-order, or daily, increments. The vertical lineations are dentinal tubules. The scale bar is 0.2 mm
Table 1 summarizes the second-order increment
data collected from each specimen. The secondorder increments are interpreted as the result of a
weekly periodicity in dentinal deposition based on
three lines of evidence. First is the average thickness of second-order increments. In 13 tusks of
Mammut, Mammuthus, and Elephas that have
second-order increments with a fortnightly period,
the average increment thickness is 0.197 mm
( Koch, 1989). As indicated in Table 1, the average
second-order increment thickness in the seven
Gomphotherium specimens analyzed here ranges
339
Table 1
Second-order increment thickness data for specimens analyzed
Specimen
F:AM 129673
F:AM 129672
F:AM 129671
F:AM 25729
F:AM 99059
F:AM 129670
UNSM 88511
Age
E.
E.
L.
L.
L.
L.
L.
Hemphillian
Hemphillian
Clarendonian
Clarendonian
Barstovian
Barstovian
Barstovian
Increment thicknessa
Yearsc
Number
Meanb
S.D.
210
244
273
175
239
350
237
0.091
0.093
0.082
0.098
0.111
0.085
0.095
0.039
0.034
0.037
0.036
0.044
0.027
0.027
4.0
4.7
5.2
3.4
4.6
6.7
4.6
a Number of increments measured
b Mean thickness (mm) of increments, measured as the distance between successive dark bands of second-order increments.
c Calculated by dividing the number of second-order increments, which represent weeks, by 52.
from 0.082 to 0.111 mm, and the average for all
seven is 0.094 mm, almost half that of the other
species. Next, second-order increments typically
contain about seven third-order increments, with
variation in number due probably to both preservation and inherent variability in the signals
responsible for increments at both scales.
Assuming that the third-order increments represent
days (based on their size and analogy with other
proboscideans), second-order increments typically
represent intervals of 1 week. Perhaps the most
significant evidence for weekly periodicity is the
number of second-order increments that occur
within the recognizable first-order features. If these
features do represent weeks, then there should be
approximately 52 per first-order feature. The thicknesses of the two first-order increments in F:AM
129673 ( Fig. 5A) were measured on the sample
block with a dial caliper and their positions identified in the second-order increment data to determine the number of second-order increments
identified within each first-order feature. The firstorder increment marked ‘a’ in Fig. 5A includes 53
second-order increments, and that marked ‘b’ contains 49 second-order increments. Similarly, the
single first-order increment visible in F:AM 129671
(not figured; marked ‘c’ in Fig. 7A) includes 52
second-order increments. Thus, both specimens
conform to expectation and strongly support the
interpretation of second-order increments as
weekly features. The evidence for the weekly periodicity of second-order increments also corrobo-
rates the annual periodicity of the first-order
increments.
Third-order increments (Fig. 5C ) were analyzed
only to assist interpretation of the second-order
increments. Detailed analysis of these smallestscale features is complicated by the inconsistency
of their expression both along a single transect
and across a thin section. Frequently, third-order
increments are only visible in the dark portion of
the weekly increments. However, all specimens
examined in this study had recognizable thirdorder increments in all thin sections prepared. In
areas of good preservation, as in Fig. 5C, the
hierarchical relationship between second- and
third-order increments is evident.
6.3. Luminance transects
The autocorrelation results for the seven individual luminance transects analyzed are presented
in Table 2. The purpose of these autocorrelation
analyses is to evaluate the identification of the
second-order increments based on analysis of the
luminance data from the individual, discrete sampling transects and to determine whether the features marked are reasonably the same both within
and between tusks. For each transect, the mean
increment thickness is based on the identification
and measurement of second-order increments
using Optimas. The implied lag assumes that the
second-order increments were properly identified
and represents the lag expected to have statistically
340
Table 2
Results of autocorrelation of luminance transects from three specimens
Specimen
Transect
Mean increment
thickness (mm)
Implied
laga
Statistically
significant lagsa
Implied increment
thickness (mm)
F:AM 129670
5
7
10b
3
10b
2b
6b
0.090
0.083
0.079
0.107
0.069
0.123
0.086
12–13
11–12
11–12
15
9–10
17–18
12–13
10–14
9–14
10–11
15–17
9–17
17–24
11–19
0.071–0.099
0.064–0.099
0.071–0.078
0.107–0.121
0.064–0.121
0.121–0.170
0.078–0.135
F:AM 129671
F:AM 129673
a Lags are in number of increments.
b Detrended to remove a linear trend prior to autocorrelation.
significant r when the luminance data are autocort
related. The implied lag is the ratio of the mean
increment thickness measured along a given luminance transect to the luminance sampling interval,
0.0071 mm. The ranges for implied lags in Table 2
are for means that fall between the integer valued
lags. The statistically significant lags are the result
of the autocorrelation of the data for each of the
seven individual luminance transects and is determined from the values of Z ; for those transects
t
that have more than one statistically significant
peak, only that corresponding to the mean
increment thickness is included. Peaks in Z that
t
do not correspond to mean increment thickness
can occur at lags that are multiples of a base
periodicity in the data or because of other potentially periodic features in the luminance data, such
as Schreger banding. The implied increment thickness is the converse of the implied lag: it is the
product of the statistically significant lags and the
luminance sampling interval. Comparisons can be
made either between the mean and implied
increment thicknesses or between the implied and
statistically significant lags.
In six of the seven transects, the mean increment
thickness and the implied lag are in the range of
the statistically significant lags and the implied
increment thickness. Additionally, in three of these
six transects, the lag with the highest Z statistic
corresponds either to one of the values of the
implied lag (transect 7 of F:AM 129670, transect
6 of F:AM 129673) or exactly to the implied lag
(transect 3 of F:AM 129671). For the seventh
autocorrelated transect, transect 10 of F:AM
129670, the implied lag does overlap with the
statistically significant lag, and the mean increment
thickness is less than one 0.001 mm larger than
the implied thickness for t=11. Two additional
transects, not included in Table 2, have peaks in
r that are not statistically significant but do corret
spond exactly to the implied lag for those transects.
These results indicate that the features marked as
second-order increments can be objectively identified on the basis of the luminance profile given
good preservation and also imply that the ‘same’
features are consistently marked. Given that no
qualitative differences were evident between the
seven transects in Table 2 and others from those
specimens or from the remaining four specimens,
it is reasonable to conclude that second-order
increments were consistently and objectively identified, even in those individual luminance transects
that were not checked with autocorrelation.
6.4. Second-order increment thickness profiles
Measurements of second-order increment thickness are presented in Table 1 and Figs. 6–8. The
similarities in the mean and standard deviation of
increment thickness in Table 1 for all seven specimens further argue for the ‘sameness’ of the features measured. The autocorrelograms are based
on autocorrelation of the increment thickness data.
None of the Barstovian specimens has any
regular or cyclic pattern in increment thickness for
sections of tusk representing 4.6–6.7 years of tusk
341
Fig. 6. Second-order increment thickness and autocorrelograms of thickness data for late Barstovian Gomphotherium tusks. In
increment thickness plots, the abscissa begins with the oldest increment measured, at the enamel–dentin junction, and moves forward
in time toward the axis of the tusk or the pulp cavity surface, which represent the youngest increments in a section. (A) Plot of
second-order increment thickness for F:AM 99059. The asterisks mark places where cracks in the dentin could not be bridged by
luminance transects. The number of increments missing was estimated using the length of missing section and the average increment
thickness for the whole slide; thus, increment numbers for F:AM 99059 are somewhat approximate. (B) Autocorrelogram, Z statistics
and critical values of Z (a=0.05) for F:AM 99059. Increment thickness data were detrended prior to autocorrelation. (C ) Plot of
t
second-order increment thickness for F:AM 129670. (D) Autocorrelogram, Z statistics and critical values of Z (a=0.05) for F:AM
t
129670. (E ) Plot of second-order increment thickness for UNSM 88511. (F ) Autocorrelogram, Z statistics and critical values of Z
t
(a=0.05) for UNSM 88511. Increment thickness data were detrended prior to autocorrelation.
growth ( Fig. 6). The pattern for F:AM 99059
(Fig. 6A) exhibits two areas of relatively high
growth, near the beginning and again at the end
of the section plotted. However, given the inconsistency of the pattern, the simplest explanation is
simply individual variation in growth rate. This is
corroborated by the lack of a temporally significant
periodicity in the autocorrelogram in Fig. 6B.
Although the correlation coefficient for t=14 is
significant, and 14 is close to the value expected
342
Fig. 7. Second-order increment thickness and autocorrelograms of thickness data for late Clarendonian Gomphotherium tusks. (A)
Plot of second-order increment thickness for F:AM 129671. (B) Autocorrelogram, Z statistics and critical values of Z (a=0.05) for
t
F:AM 129671. (C ) Plot of second-order increment thickness for F:AM 25729. (D) Autocorrelogram, Z statistics and critical values
of Z (a=0.05) for F:AM 25729.
t
for a lunar monthly signal for the second-order
increments, the patterns in Fig. 6A and B are not
consistent overall with a regular cyclicity in the
data, and an alternative temporal signal (i.e.
weekly) for the second-order increments is well
supported. F:AM 129670 and UNSM 88511
(Fig. 6C and E) have irregularly spiky patterns
and the lowest standard deviations, indicating that
few increments stray far from the average thickness. (Increment thickness measurements on three
additional thin sections from F:AM 129670 cover
most of the life history of that animal; the
increment thickness profiles, however, are not
qualitatively different from Fig. 6C and are not
presented here.) The autocorrelograms for F:AM
129670 and UNSM 88511 ( Fig. 6D and F ) are
characteristic of an aperiodic time series. The peaks
in Z that do cross the critical values are associated
t
with relatively short lags that do not correspond
to temporal signals clearly associated with a cyclic,
sub-annual pattern of variation in increment
thickness.
Increment thickness data from one of the two
late Clarendonian specimens, F:AM 129671,
exhibit a pattern that is consistent with seasonal
growth ( Fig. 7A and B). The pattern of shading
beneath the plot of increment thickness indicates
the location and extent of the first-order increment
‘c’ discussed above, as well as the location and
extent of the light part of a second first-order
increment toward the enamel. The question mark
in the long shaded stretch after increment 96
indicates the absence of first-order increments
through that part of the section. The overall
impression of Fig. 7A is that there are a number
of areas of high growth rate separated by narrower
zones of low growth. The most prominent areas
of high growth are from increment 62 to increment
96, from 132 to about 170 and from just past 190
to about 240. A possible fourth zone of high
343
Fig. 8. Second-order increment thickness and autocorrelograms of thickness data for early Hemphillian Gomphotherium tusks. (A)
Plot of second-order increment thickness for F:AM 129673. (B) Autocorrelogram, Z statistics and critical values of Z (a=0.05) for
t
F:AM 129673. (C ) Plot of second-order increment thickness for F:AM 129672. (D) Autocorrelogram, Z statistics and critical values
of Z (a=0.05) for F:AM 129672.
t
growth extends from increments 21 to 56, which
would have a corresponding zone of slow growth
from increment 1 to 20. The two peaks at
increments 5 and 12 are possibly erroneously large
because the quality of the dentin near the enamel–
dentin junction is often different, and increments
there are more difficult to identify. A final area of
high growth seems to commence at the end of the
plot for F:AM 129671; these peaks are generally
smaller than those in other zones of high growth
as increments near the axis are usually somewhat
thinner and often also more difficult to identify.
Three of the complete zones of high growth seem
to last about 35–40 weeks. The only exception to
this is the zone from increments 190 to 240. The
zones of high growth from increments 21 to 56
and from 62 to 96 roughly correspond to the
position of the light portions of the first-order
increments.
The pattern of growth exhibited by F:AM
129671 seems to be periodic but does not precisely
correspond to annual cycles in that pairs of high
and low growth zones do not come in intervals of
52 second-order increments. This is supported by
the autocorrelogram ( Fig. 7B), the overall pattern
of which is indicative of cyclicity in the data, but
the peak in significance is for t=57–63 (and again
at t=65), too long to be annual if all secondorder increments are properly identified. However,
the autocorrelation coefficient for t=53
(Z =1.90) is marginally significant. Also, the stat
tistically significant negative values of r in Fig. 7B,
t
which result from cyclic data lagged so that it is
perfectly out of phase with itself, range from t=
24 to 37. This range includes t=26, which is the
lag associated with an annual cycle (52 weekly
increments) that is out of phase with itself. A
variety of factors could lead to an inexact correspondence of the identified cycles to annual cycles,
including differences in the behavioral and physio-
344
logical responses of the animal from year to year,
annual climate variability, and error in increment
identification. However, the five identifiable zones
of high growth imply approximately 5 years of
growth in Fig. 7A, and the number of increments
indicates that there should be just over 5 years
represented in this section of the tusk ( Table 1).
Thus, a reasonable conclusion is that this specimen
is exhibiting a pattern of seasonal growth in which
long periods of high growth are interspersed with
somewhat shorter intervals of slower growth,
although these periods do not seem to correlate
perfectly with annual cycles.
Overall, the other late Clarendonian specimen,
F:AM 25729, does not appear to have a seasonal
growth pattern (Fig. 7C and D). However, the
first 90 or so increments are somewhat suggestive
of a pattern like that of F:AM 129671. The first
34 increments are a relatively high growth period
and are followed by 10 weeks of slower growth
beginning with increment 35. Another high growth
period commences with increment 45 and lasts
until about increment 80, or 35 weeks. The remainder of the increments represent almost 2 years of
lower growth without an identifiable pattern, and
none of the increments over this period is as thick
as the thickest increments in either of the earlier
high growth periods. It is possible that the first
part of this profile, up to increment 80, represents
one full seasonal cycle and the high growth period
of another. However, in the absence of clear firstorder increments or a more consistent growth
pattern, this explanation is difficult to evaluate.
The autocorrelogram has a single significant peak
at t=35, which could be influenced by the pattern
noted for the first 80 increments. Otherwise, the
autocorrelogram does not indicate any strong
cyclic behavior in the data.
As with the Clarendonian specimens, only one
of the early Hemphillian specimens, F:AM 129673,
has a pattern that is interpretable as the result of
seasonal growth (Fig. 8A and B). The shading
indicates the position of the first-order increments
discussed above, and the question mark again
indicates the portion of the sample for which there
is no evidence of first-order increments. Two prominent areas of high growth run from increment 83
to 105 and from increment 134 to 148. The first
of these zones corresponds closely to the position
of the dark portion of first-order increment ‘a’
( Fig. 8A), which runs from increment 89 to 95 in
Fig. 8A. The other zone of high growth is slightly
broader than the dark portion of first-order
increment ‘b’ (Fig. 5A), which only runs from
about increment 137 to 143. However, the placement of the first-order increments is measured with
dial calipers and hence is somewhat gross relative
to the precision of the increment thickness data.
Two other zones of high growth can also be
identified. The more distinct of these is from
increment 178 to increment 195. Although there
are two peaks just beyond this zone, both are close
to the axis of the tusk where increments are more
difficult to identify, and so each may result from
inaccurate identification of increments. The
second, less distinct zone of high growth is from
increment 32 to 43. This zone would be more
pronounced but for single peaks on either side at
increments 26 and 52. Both of these increments
are about twice as thick as the surrounding
increments, and it is possible that they are incorrectly marked. Beginning with the zone of high
growth at increment 32, the pairs of high and low
growth zones have a fairly close correspondence
to intervals of 52 increments, as expected for a
seasonal pattern of growth. The four zones of high
growth separated by zones of low growth imply
that this section represents about 4 years, the same
number of years inferred from the increment count
( Table 1). Additionally, the autocorrelogram
( Fig. 8B) is consistent with an annual periodicity
as r is statistically significant for t=43–53, though
t
the highest r is for t=46, a discrepancy that could
t
easily result from variability in tusk growth and a
small number of improperly marked increments.
The other Hemphillian specimen, F:AM 129672
( Fig. 8C and D), has little evidence of a seasonal
pattern. Some intervals of relatively low growth
are evident, such as from increment 7 to 20 and
again around increment 125, but these are not
particularly distinct and do not relate to welldefined high growth zones. The most notable
aspect of the profile is the extended period of very
low growth from increment 195 to the end of the
plot, an interval of just more than a year. The
increments over that section are consistently thin-
ner than almost all of the preceding increments,
and they represent an interval that is conspicuously
different from the earlier portion of the profile.
7. Discussion
The increment thickness profiles generally correspond to the expectations of an increase in seasonality during the late Miocene. However, the lack
of conformity within both the Clarendonian and
Hemphillian samples and the small sample size of
the study suggest caution in interpreting the data.
The lack of seasonal patterns in F:AM 129672 and
F:AM 25729 may result from interannual or geographic variation in the degree of seasonality.
Some late Pleistocene mammoths, such as those
from the Dent site in Colorado, do not exhibit a
high-amplitude variation in second-order growth
increments, despite clearly seasonal oxygen isotope
profiles ( Fisher, 1995). Additionally, the sample
of tusks may contain a range of ages and a mix
of tusks from males and females. Patterns of tusk
growth do change with age, sex and reproductive
status ( Fisher, 1996). Thus, a lack of strong patterns in two of the four more recent specimens
does not necessarily invalidate the observed patterns of the remaining specimens. Notable in this
regard is that the only specimens that have clear
first-order increments, associated with seasonality
in extant mammals ( Klevezal and Kleinenberg,
1969), are also the only specimens to exhibit
seasonal patterns of growth in the second-order
increments.
A question that remains is whether the late
Miocene was characterized by seasonality in temperature, precipitation, or both. A slight difference
in the second-order increment patterns of F:AM
129671 and F:AM 129673 is suggestive of an
answer. The lengths of the three most prominent
high growth intervals in F:AM 129671, the late
Clarendonian specimen, range from 35 to
40 weeks, and the low growth periods are briefer
(Fig. 7A and C ). This pattern is similar to that of
many late Pleistocene tusks, which commonly have
high growth throughout most of the year and a
couple of months of slow growth. As discussed,
this pattern in Pleistocene tusks is interpreted to
345
result from nutritional stress associated with highly
seasonal temperature distributions (Fisher, 1988;
Koch, 1989), which is corroborated by the pattern
of oxygen isotope variation in the tusk dentin
( Koch et al., 1989). The high growth intervals in
F:AM 129673, the late Hemphillian specimen, are
only 11–24 weeks long ( Fig. 8A), essentially the
inverse of the late Clarendonian and late
Pleistocene patterns. This implies that the growing
season may have been much shorter and more
distinct in the early Hemphillian than in the late
Clarendonian. A possible explanation for the
difference between late Clarendonian and early
Hemphillian tusks is the gradual increase in aridity
and the development of a single, distinct wet
season. Seasonally abundant precipitation could
lead to brief periods of high food abundance and
high growth rates in proboscideans.
8. Conclusion
This study had two main goals. The first was
to describe the growth and structure of
Gomphotherium tusks and to make comparisons
with the tusks of other proboscideans. The specimens examined indicate that gomphotheres are
similar to other proboscideans in that their tusks
grew throughout life and are composed of incremental features that occur on multiple scales, both
spatially and temporally. Annual and daily
increments are similar among all species examined
thus far, but gomphotheres are similar to some
specimens of Mammuthus (unpub. data) in having
intermediate-scale increments that have a weekly,
rather than fortnightly or lunar monthly, period.
The second goal was to use patterns of tusk growth
to test the hypothesized increase in seasonality
during the late Miocene in North America. The
increment thickness profiles correspond to the
expectations of an increase in seasonality in that
only late Clarendonian and Hemphillian tusks
have seasonal patterns of growth. Additionally,
the differences between the late Clarendonian and
Hemphillian specimens are consistent with the
development of a wet season and an increase in
the seasonality of precipitation. Unsurprisingly,
the results also suggest that the changes in climate
346
may have been more complicated than a simple
secular increase in seasonality. Regardless, the data
presented here do support an increase in seasonality, and hence lend support to the climate change
hypothesis for mammalian faunal changes in
North America during the late Miocene.
The interpretation of the growth patterns can
be tested in three ways. The first is to increase the
sample size. A number of the localities represented
in this study have numerous isolated tusks; analysis
of multiple specimens from the same locality would
be important to demonstrate the generality and
replicability of the patterns noted here. Next, tusks
of extant elephants from a range of climatic
regimes could be analyzed to determine the impact
of different patterns of seasonality on tusk growth
in a modern analog. For example, a comparison
of tusks of elephants from the grasslands of East
Africa with those of elephants from the forests of
Central Africa could be useful in determining the
effect of aridity and seasonal precipitation on tusk
growth. The third test would be to examine the
patterns of variation in stable isotope composition
in a manner analogous to that of Koch et al.
(1989). If temperature, through its effect on the
availability of food resources, is a major factor in
the changes in growth rate, the variation in oxygen
isotope composition of tusk hydroxyapatite should
correlate with the variation in increment thickness.
Variation in the carbon isotope composition within
individual tusks could be used to investigate
changes in diet that result from shifting food
resource abundances on annual time scales.
Carbon isotope measurements of tusks from
middle to latest Miocene localities might indicate
long-term trends in diet that reflect habitat change
over the course of Miocene in response to climate
change. The carbon isotope composition of the
teeth of Miocene herbivorous mammals from
North America and elsewhere has indicated an
increase in the abundance of C plants ( lowland
4
and arid grasses) relative to C plants (trees,
3
shrubs, herbs), both of which have distinct carbon
isotope signatures (Cerling et al., 1997). Thus, if
the type of vegetation available to gomphotheres
were changing over the course of each year of an
individual’s life or over the course of the late
Miocene in response to changing climate and
habitat, the shift might be detectable from the
carbon isotope composition of the tusks. These
tests, some of which are in progress, will serve to
increase our understanding of the causes and
dynamics of the climatic and ecological changes
that occurred in North America as grassland habitats spread out over the last 10 million years of
the Miocene.
Acknowledgements
This paper would not have been possible without the generous access to specimens provided by
R. Tedford (AMNH ) and M. Voorhies ( UNSM ).
The research benefited greatly from the guidance
of D.C. Fisher. The manuscript was improved by
the comments and advice of C. Badgley, J. Bloch,
D.C. Fisher, G. Gunnell, E. Kowalski, B. Sanders,
J. Trappani, and M. Uhen, though none of them
should be held accountable for its final form. P.
Koch and J.F. Thackeray provided helpful reviews.
This research was supported by NSF grant
SBR-9211984 to D.C. Fisher.
References
Alroy, J., 1992. Conjunction among taxonomic distributions
and the Miocene mammalian biochronology of the Great
Plains. Paleobiology 18, 326–343.
Barry, J.C., Johnson, N.M., Raza, S.M., Jacobs, L.L., 1985.
Neogene mammalian faunal change in southern Asia: correlations with climatic tectonic and eustatic events. Geology
13, 637–640.
Boyde, A., 1979. Carbonate concentration crystal centers core
dissolution caries cross striations circadian rhythms and
compositional contrast in the SEM. J. Dent. Res. 58,
981–985.
Burke, A., Castanet, J., 1995. Histological observations of
cementum growth in horse teeth and their application to
archaeology. J. Archaeol. Sci. 22, 479–493.
Cerling, T.E., Harris, J.M., MacFadden, B.J., Leakey, M.G.,
Quade, J., Eisenmann, V., Ehleringer, J.R., 1997. Global
vegetation change through the Miocene/Pliocene boundary.
Nature 389, 153–158.
Corner, R.G., 1976. An early Valentinian vertebrate local fauna
from southern Webster County, Nebraska. Masters thesis,
University of Nebraska.
Davis, J.C., 1986. Statistics and Data Analysis in Geology.
second ed., Wiley, New York.
Dean, M.C., 1987. Growth layers and incremental markings in
hard tissues, a review of the literature and some preliminary
observations about enamel structure in Paranthropus boisei.
J. Hum. Evol. 16, 157–172.
Fisher, D.C., 1984. Taphonomic analysis of late Pleistocene
mastodon occurrences: evidence of butchery by North
American Paleo-Indians. Paleobiology 10, 338–357.
Fisher, D.C., 1987. Mastodon procurement by Paleoindians of
the Great Lakes Region: hunting or scavenging? In: Nitecki,
H., Nitecki, D.V. (Eds.), The Evolution of Human Hunting.
Plenum Press, New York, pp. 309–421.
Fisher, D.C., 1988. Season of death of the Hiscock mastodonts,
in: Laub, R.S., Miller, N.G., Steadman, D.W. (Eds.), Late
Pleistocene and Early Holocene Paleoecology and Archaeology of the Eastern Great Lakes Region. Bulletin of the
Buffalo Society of Natural Sciences Vol. 33., 115–125..
Fisher, D.C., 1990. Age, sex and season of death of the
Grandville mastodon. Michigan Archaeol. 36, 141–160.
Fisher, D.C., 1995. in: Season of Death of the Dent mammoths,
Society of American Archaeologists, Abstracts for 60th
Annual Meeting, 96.
Fisher, D.C., 1996. Extinction of proboscideans in North America. In: Shoshani, J., Tassy, P. (Eds.), The Proboscidea.
Oxford University Press, Oxford, pp. 296–315.
Flower, B.P., Kennett, J.P., 1994. The middle Miocene climatic
transition: East Antarctic ice sheet development, deep ocean
circulation and global carbon cycling. Palaeogeogr. Palaeoclimatol. Palaeoecol. 108, 537–555.
Fox, D.L., Fisher, D.C., 1994. Tusk growth rate in Loxodonta
africana as recorded by incremental laminae in tusk dentin.
J. Vert. Paleontol. 14, 26A.
Gysi, A., 1931. Metabolism in adult enamel. Dent. Digest 37,
661–668.
Halberg, F., 1983. Quo vadis basic and clinical chronobiology,
promise for health maintenance. Am. J. Anat. 168, 543–594.
Haq, B.U., Hardenbol, J., Vail, P.R., 1987. Chronology of fluctuating sea levels since the Triassic. Science 235, 1156–1167.
Hewer, H.R., 1964. The determination of age, sexual maturity,
longevity and a life-table in the grey seal (Halichoerus
grypus). Proc. Zool. Soc. Lond. 142, 593–634.
Janis, C.M., 1989. A climatic explanation for patterns of evolutionary diversity in ungulate mammals. J. Palaeontol. 32,
463–481.
Kimura, F., 1939. Horoshitsu ni mirareru Seicho-sen no shuki.
Kobyo-shi 13, 454–455.
Klevezal, G.A., Kleinenberg, S.E., 1969. Age Determination of
Mammals from Annual Layers in Teeth and Bones. Israel
Program for Scientific Translations, Jerusalem. translated
from Russian.
Klevezal, G.A., 1980. Layers in the hard tissues of mammals
as a record of growth rhythms of individuals. In: Perrin,
W.F., Myrick, A.C. ( Eds.), Age Determination of Toothed
Whales and Sirenians. Reports of the International Whaling
Commission. Cambridge, Special Issue 3, pp. 89–94.
Klevezal, G.A., Mina, M.V., 1990. Daily layers and hibernation
marks in incisor dentin of Sicista pseudonapaea and some
biological remarks. Acta Theriol. 35 3–4, 345–356.
347
Koch, P.L., 1989. Paleobiology of Late Pleistocene Mastodonts
and Mammoths from Southern Michigan and Western New
York, Ph.D. thesis, University of Michigan.
Koch, P.L., Fisher, D.C., Dettman, D.L., 1989. Oxygen isotope
variation in the tusks of extinct proboscideans: a measure
of season of death and seasonality. Geology 17, 515–519.
Laws, R.M., 1952. A new method of age determination for
mammals. Nature 169, 972.
Laws, R.M., 1962. Age determination of pinnipeds with special
reference to growth layers in teeth. Zeitschrift fur Säugetierkunde 27, 129–146.
Lieberman, D.E., 1993. The rise and fall of seasonal mobility
among hunter–gatherers. Curr. Anthropol. 34, 599–631.
Lieberman, D.E., 1994. The biological basis for seasonal
increments in dental cementum and their application to
archaeological research. J. Archaeol. Sci. 21, 525–539.
Marsh, H., 1980. Age determination of the dugong (Dugong
dugon (Müller)) in northern Australia and its biological
implications. In: Perrin, W.F., Myrick, A.C. (Eds.), Age
Determination of Toothed Whales and Sirenians. Reports
of the International Whaling Commission, Cambridge,
Special Issue 3, pp. 181–200.
Mayhew, D.F., 1978. Age structure of a sample of subfossil
beavers (Castor fiber L). In: Butler, P.M., Joysey, K.A.
( Eds.), Development, Function and Evolution of Teeth.
Academic Press, London, pp. 495–505.
Miani, A., Miani, C., 1972. Circadian advancement rhythm of
the calcification front in dog dentin. Panminerva Medica
14, 127–136.
Miller, K.G., Fairbanks, R.G., Mountain, G.S., 1987. Tertiary
oxygen isotope synthesis sea-level history and continental
margin erosion. Paleoceanography 2, 1–19.
Mummery, J.H., 1924. The Microscopic and General Anatomy
of the Teeth, Human and Comparative. Oxford University
Press, Oxford. 240 pp.
Okada, M., 1943. Hard tissues of animal body. The Shanghai
Evening Post., 15–31. date unknown.
Owen, R., 1840–1845. Odontography. Hippolyte Bailliere,
London.
Perrin, W.F., Myrick, A.C., 1980. Age Determination of
Toothed Whales and Sirenians. Reports of the International
Whaling Commission. Cambridge. Special Issue 3, 229 pp.
Rosenberg, G.D., Simmons, D.J., 1980. Rhythmic dentinogenesis in the rabbit incisor: circadian, ultradian, and infradian
periods. Calcif. Tiss. Int. 32, 29–44.
Saxon, A., Higham, C., 1969. A new research method for economic prehistorians. Am. Antiq. 34, 303–311.
Schour, I., Hoffman, M.M., 1939. Studies in tooth development
I: the 16 microns calcification rhythm in the enamel and
dentin from fish to man. J. Dent. Res. 18, 91–102.
Schmidt, W.J., Kiel, A., 1971. Polarizing Microscopy of Dental
Tissues. Pergamon Press, Oxford.
Sheffer, V.B., 1950. Growth layers on the teeth of Pinnepedia
as an indicator of age. Science 112, 309–311.
Sheffer, V.B., 1970. Growth layers in a dugong tooth.
J. Mammal. 51, 187–190.
348
Singh, G., 1988. History of aridland vegetation and climate: a
global perspective. Biol. Rev. 63, 156–196.
Slooten, E., 1991. Age, growth and reproduction in Hector’s
dolphin. Can. J. Zool. 69, 1689–1700.
Stucky, R.K., 1990. Evolution of land mammal diversity in
North America during the Cenozoic, in: Genoways, H.
( Ed.), Current Mammalogy Vol. 2. Plenum, New York,
pp. 375–432.
Swan, A.R.H., Sandilands, M., 1995. Introduction to Geological Data Analysis. Blackwell Science, Oxford. 446 pp
Tedford, R.H., Skinner, M.F., Fields, R.W., Rensberger, J.M.,
Whistler, D.P., Galusha, T., Taylor, B.E., Macdonald, J.R.,
Webb, S.D., 1987. Faunal succession and biochronology of
the Arikareean through Hemphillian internal (Late Oligocene through Earliest Pliocene epochs) in North America.
In: Woodburne, O. ( Ed.), Cenozoic Mammals of North
America. University of California Press, Berkeley, CA,
pp. 153–210.
Tobien, H., 1972. Status of the genus Serridentinus Osborn 1923
(Proboscidea, Mammalia) and related forms. Mainzer Geowissenschaftliche Mitteilungen 1, 143–191.
Webb, S.D., 1977. A history of savanna vertebrates in the new
world — Part 1: North America. Annu. Rev. Ecol. Syst.
8, 355–380.
Webb, S.D., 1984a. Ten million years of mammal extinctions
in North America. In: Martin, P.S., Klein, R.G. ( Eds.),
Quaternary Extinctions: A prehistoric revolution. The University of Arizona Press, Tucson, AZ, pp. 189–210.
Webb, S.D., 1984b. On two kinds of rapid faunal turnover. In:
Berggren, W.A., van Couvering, J. ( Eds.), Catastrophes and
Earth History: The New Uniformitarianism. Princeton University Press, Princeton, NJ, pp. 417–436.
Wei, K.Y., Kennett, J.P., 1986. Taxonomic evolution of Neogene planktonic foraminifera and paleoceanographic relations. Paleoceanography 1, 67–84.
Wilkinson, L., 1990. SYSTAT: The System for Statistics. Systat,
Evanston, IL.
Wolfe, J.A., 1985. Distribution of major vegetational types
during the Tertiary, in: The Carbon Cycle and Atmospheric
CO Geophysical Monograph Vol. 32., 357–375.
2
Woodburne, M.O., 1987. Cenozoic Mammals of North America. University of California Press, Berkeley, CA.
Woodruff, F., Savin, S.M., 1989. Miocene deepwater circulation. Paleoceanography 4, 87–140.
Wright, J.D., Miller, K.G., Fairbanks, R.G., 1992. Early and
middle Miocene stable isotopes: implications for deepwater
circulation and climate. Paleoceanography 7, 357–389.

Growth increments in Gomphotherium tusks and implications for late

Transcription

Similar documents

Credit Card Payment Form

2015 SCWTCA Wheaten Boutique Reservation Form

EZ Ancor Hollow Door downloadable

QT 2000 - Edelstahl-Service

PDF Marco LM-600 Brochure

Medrad Mark 7 Arterion Brochure

NATURAL REFLECTIONS

huvitz auto ref-keratometer hrk-8000a