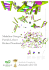Drawing - Edutopia

Drawing
Art
into the
equation
Aesthetic computing gives math a clarifying
visual dimension.
BY JENN SHREVE
T
eaching math at
a high-risk juvenile detention
facility in Marion
County, Florida,
Debra Hamed
was more used to having assignments crumpled up than eagerly completed. Then she
heard about something with a slightly puzzling name: aesthetic computing.
Aesthetic computing is a curriculablending approach that applies the theory
and practice of art to computing and problem solving. Hamed tried it in her History of
Math Class. Following the aesthetic method,
Hamed asked the students to create a piece of
artwork or a poem that conveys the ideas
behind key mathematical pioneers—people
such as Pythagoras, Ptolemy, Leonardo da
Vinci, and Fibonacci.
Hamed was amazed at the results.
The students not only grasped the innovations they were illustrating, but “because
they were successful,” Hamed adds, “it provided them with enthusiasm when they saw
the same concepts in the textbook.”
Of course, you don’t have to go to a juvenile detention center to find students with a
strong aversion to math. Many teens and preteens struggle to make sense of geometry
and the abstract symbols, terms, and logic of
algebra.And because they don’t see the subject as relevant, students say they have little
42
EDUTOPIA JULY/AUGUST 2006
motivation to figure it out.“‘When will I ever
use this?’That’s their mantra.They just don’t
see any need for it,” says Bunny McHenry, a
Marion County high school teacher who
attended the workshop with Hamed.
In the Los Angeles Unified School District
alone, 44 percent of ninth graders enrolled in
beginning algebra during the fall 2004 semester failed. Because passing the course is
required for graduation, many take it and
flunk again. With each failing grade, a student’s motivation and prospects for earning a
diploma get dimmer.
Aesthetic computing attempts to reach
those frustrated by traditional math instruction by presenting abstract mathematical
concepts in a more creative and personal
way. Students break down difficult mathematical concepts, such as algebraic equations, into their basic parts, figure out how
those parts relate to one another, then recreate the equation creatively. For example,
a standard equation for graphing lines on a
slope such as y = mx + b might become a
hamburger, with y representing the whole
burger, m referring to the meat, and x standing in for spices. Multiplication is indicated
by the fact that the meat and spices are
mixed together, and b is added to represent
hamburger buns.
Students then write a story about the
burger or draw a picture of it. (See “Five
Easy Steps to Aesthetic Computing,” page 43.)
Not only does the process enable students
to understand the equation in a more meaningful way, the art and stories they create
can later guide and inspire them when they
need to solve the same equations using standard notation later on.
This type of visual approach is not only
fun but also increasingly necessary in a
world in which everybody needs to have
some basic understanding of mathematical
concepts—not just to graduate but to thrive
after graduation.“Students learn in lots of different ways,” says Cathy Seeley, president of
the National Council of Teachers of
Mathematics. In fact, representing mathematical concepts in multiple forms is among the
NCTM’s five content standards for teaching
K–12 math. According to the NCTM,
“Representations are necessary to students’
understanding of mathematical concepts
and relationships. Representations allow
students to communicate mathematical
approaches, arguments, and understanding
to themselves and to others.They allow students to recognize connections among
related concepts and apply mathematics to
realistic problems.”
Accessible Arithmetic
Paul Fishwick, the University of Florida
computer science professor who developed
the aesthetic-computing method, says his
inspiration came from popular culture. He
DIY
wanted to do for math what the 1982 Disney
film Tron did for computer science.With its
then–cutting edge graphics and its actionheavy plot about a hacker overriding a
malevolent operating system, it made a previously dry and impenetrable field of study
visual and exciting.“If we have the capability of doing some of these fantastic things
with technology, such as the immersive environments and 3-D graphics for entertainment
purposes, why are we still programming and
doing math by scratching with pencil on
paper, much the same way we did 2,000 years
ago?” Fishwick asks.
Surprisingly, aesthetic computing requires
no expensive software or even a computer.
It’s so flexible, in fact, that it can be used just
as effectively for learning basic middle school
prealgebra as university-level programming
courses. Says Jodee Rose, a former art and
math teacher who developed a middle school
lesson plan for teaching the method,“It’s low
tech, but it’s high tech ideas, because it’s
working through computer language, which
kids are going to need to learn eventually.”
Rose, who lost her job when her school’s art
department budget was cut, hopes it might
provide an opportunity for kids to create art
as part of an academic discipline.
Reaching students who aren’t naturally
drawn to math, whether they’re phobic or
simply more artistically inclined, isn’t the
only benefit of the method. Fishwick notes
that aesthetic computing “provides a continuum for the constructivist, concrete-object
approach to mathematics” many teachers use
in the early grades—for example, using three
apples to explain the number three.
Unfortunately, he says, this approach typically
stops after fourth or fifth grade.
For Katrina Indarawis, who developed a
high school lesson plan for aesthetic computing, it’s a less intimidating way of introducing
new concepts in math. “I thought it would
really help students not be so scared of some
of the math notations,” she says.“It helps them
break it down and turn it into something they
like.”Obviously, aesthetic computing is no
cure-all.“You still have to teach the same concepts, but it may reinforce those concepts,
because they’re using it in a different way,”
says McHenry. She plans to try the method in
her classroom during the next school year.
The New Math
The aesthetic-computing method is still new.
No formal studies have confirmed its usefulness. Nor is there much in the way of materials that would help teachers who want to try
it out. Hoping to address these problems,
Fishwick is trying to attract more researchers
to study the method and to raise money to
put on additional workshops.
For teachers who don’t want to wait, the
lesson plans developed by Rose and
Indarawis, thanks to a National Science
Foundation grant, are now available through
Fishwick’s Web site, as are several articles on
aesthetic computing, links to free software
developed by students of Fishwick, and a
discussion area. In addition, MIT Press is
publishing Aesthetic Computing, a book
Fishwick contributed to that discusses the
more theoretical aspects of the method. He’s
Five Easy Steps to
Aesthetic Computing
1. Students begin by breaking down the
mathematical expression they’re working
on into an “expression tree.” Like a sentence diagram, it helps them understand
the components of an expression—in this
case, individual parts of the equation,
what each one does, and how they relate
to one another.
2. Based on their diagram, students
make a list of all the parts of the equation
(constants, variables, and operations) as
well as hidden meanings such as the
“argument of” relationship (for example,
1 and 2 are arguments of + in the expression “1 + 1 = 2”).
3. They then brainstorm ideas they’d like
to use to reexpress the elements of the
expression tree creatively. Once they’ve
chosen a theme, they come up with a
means to express it—for example, as a
drawing, a story, or a sculpture.
4. Now it’s time to put things together. The
parts of the equation listed in step 2 are
paired with the creative objects brainstormed in step 3, keeping in mind that the
metaphor needs to function in the same
way as the equation. So, for example, two
objects that are multiplied would be combined, just like meat and spices. Once students have worked these details out, they
can use their list (from step 2) to make a
key, linking the parts of the equation with
their language counterparts (“y = meat; x =
spices”).
5. When the standard equation and its
more creative expression are mapped out,
it’s time to build the new, creative version.
From lesson plans found in Paul Fishwick’s report
“Introduction to the Aesthetic Computing Method for
Teaching Algebra in Middle and High School.”
also working on a more advanced computer
tool that helps students simulate the process
(and a student of his is developing an academic video game that will use these methods
and problem-solving tools)—anything, he
says, to help get more kids to make a personal connection to math.
“Now that we’re recognizing how
important math is, we have to expand the
ways we teach so we can allow people who
learn in different
ways
or
have HOT LINKS
web links about aesstrengths in differ- For
thetic computing, go to
ent areas to have www.edutopia.org/1577
entry points into
math,” says the NCTM’s Seeley. Fishwick
believes aesthetic computing could grow
into one of those entry points.
Debra Hamed, for one, is a true believer:
“I’m fired up to teach math again.”d
Jenn Shreve wrote “No Train, No Gain” in our
November 2005 issue.
2006 JULY/AUGUST EDUTOPIA
43