Full text - terrapub

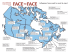
Journal of Oceanography, Vol. 55, pp. 327 to 345. 1999
A Numerical Modeling of the Upper and the Intermediate
Layer Circulation in the East Sea
CHEOL-HO KIM1 and JONG-HWAN YOON2
1Korea
Ocean Research & Development Institute, Ansan P.O.Box 29, Seoul 425-600, Korea
Institute for Applied Mechanics, Kyushu University, Kasuga, Fukuoka 816, Japan
2Research
(Received 7 September 1998; in revised form 1 December 1998; accepted 10 December 1998)
Circulation in the upper and the intermediate layer of the East Sea is investigated by using
a fine resolution, ocean general circulation model. Proper separation of the East Korean
Warm Current from the coast is achieved by adopting the isopycnal mixing, and using the
observed heat flux (Hirose et al., 1996) and the realistic wind stress (Na et al., 1992). The
simulated surface circulation exhibits a remarkable seasonal variation in the flow
patterns of the Nearshore Branch, the East Korean Warm Current and the Cold
Currents. East of the Oki Bank, the Nearshore Branch follows the isobath of shelf
topography from late winter to spring, while in summer and autumn it meanders offshore.
The Nearshore Branch is accompanied by cyclonic and anticyclonic eddies in a fully
developed meandering phase. The meandering and the eddy formation of the Nearshore
Branch control the interior circulation in the Tsushima Current area. A recirculation
gyre is developed in the region of the East Korean Warm Current in spring and grown up
to an Ulleung Basin scale in summer. A subsurface water is mixed with the fresh surface
water by winter convection in the northeastern coastal region of Korea. The well-mixed
low salinity water is transported to the south by the Cold Currents, forming the salinity
minimum layer (Intermediate Water) beneath the East Korean Warm Current water.
The recirculation gyre redistributes the core water of the salinity minimum layer in the
Ulleung Basin.
distributions. On account of strong variability in the TC,
however, and lack of direct current measurements different
views on the flow patterns have been proposed for the TC:
branching path, meandering path, and combination of both
(for schematic diagrams, refer to Kawai (1974), Naganuma
(1977) and Kim and Yoon (1996)). In an effort to explain TC
pattern suggested from the observations, a series of numerical
works have been made in 1980’s and the branching
mechanisms of the TC have been suggested using relatively
simple numerical models (Yoon, 1982a, b; Kawabe, 1982).
Since then, many new findings have been added to the
East Sea circulation: for example, presence of mesoscale
eddies in the region off the Japanese coast and in the Ulleung
Basin (Beardsley et al., 1992; Lie et al., 1995), intermediate
water characterized by the salinity minimum and the oxygen
maximum below the TC water (Kim and Chung, 1984; Kim
et al., 1991), southeastward deep current along the Korean
coast (Lie et al., 1989), subsurface countercurrent near the
Japanese coast (Hase et al., 1996) and energetic deep currents in the Japan Basin (Takematsu et al., 1996). Several
numerical experiments have been undertaken in recent years
to simulate these observed features in the East Sea (Yoon,
1991a, b; Seung and Kim, 1993; Holloway et al., 1995; Bang
1. Introduction
The East Sea is a marginal sea located in the Northwest
Pacific. Its north-south scale is more than 1000 km, extending
from 35°N to 50°N and the east-west scale is almost the
same order. The bottom topography (Fig. 1) is characterized
by the three deep basins, and the narrow continental shelves
with steep slopes: the Japan Basin to the north with depth
deeper than 3500 m, the Ulleung Basin and the Yamato
Basin to the south with depths of 2000 m. The East Sea is
connected with the surrounding seas through the very shallow
straits: the Korea/Tsushima Straits connecting with the
Yellow and the East China Seas, the Tsugaru and the Soya
Straits with the Northwest Pacific, and the Mamiya (Tartar)
Strait with the Okhotsk Sea. Flows in the straits are mostly
unidirectional and the water exchange with the North Pacific
is confined only to subsurface layers. The southern half of
the upper layer is filled with warm waters due to the
Tsushima Current (hereafter TC) flowing into the East Sea,
and the warm waters are bordered by the northern cold
waters forming the Polar Front at about 40°N.
Historically most of the hydrographic observations had
been made in the warm water region and a lot of efforts have
been made to find the path of the TC from the tracer
327
Copyright  The Oceanographic Society of Japan.
Keywords:
⋅ Tsushima Current,
⋅ East Korean Warm
Current,
⋅ nearshore branch,
⋅ separation of
western boundary
current,
⋅ intermediate water.
that results in a serious change in the oceanic condition of
the northwestern East Sea.
This study aims to simulate the circulation in the upper
and the intermediate layer in the East Sea featured by
mesoscale eddies and thermohaline processes. To simulate
the circulation more realistically we elaborated to refine the
model in various aspects. In the following sections we will
describe the configuration of the model, and then briefly
compare the simulated tracer fields with the observations.
Discussions will be focused on the seasonal behaviors of the
warm and cold currents. Finally some conclusions will be
drawn.
Fig. 1. Topography of the East Sea. (JB: Japan Basin, UB:
Ulleung Basin, YB: Yamato Basin, YR: Yamato Rise, EKB:
East Korean Bay, KP: Korean Plateau, OS: Oki Spur (Oki
Bank), NP: Noto Peninsula, K/TS: Korea/Tsushima Straits,
TS: Tsugaru Strait, SS: Soya Strait, MS: Mamiya Strait
(Tartar Strait)).
et al., 1996). Some of them were successful in simulating the
general features of the circulation to a considerable extent,
but there are still some serious defects such as overshooting
of the East Korean Warm Current (EKWC), lack of
resolvability for mesoscale variabilities and incomplete
formation of the salinity minimum water in the intermediate
layer.
The overshooting of the western boundary current is a
chronic problem in the simulation of a large scale ocean
circulation using ocean general circulation models (OGCMs)
(Bryan and Holland, 1989; Ezer and Mellor, 1992;
Cherniawsky and Holloway, 1993). Getting a proper separation latitude of the western boundary current is the key
process in OGCM to simulate the currents and water masses
correctly in the northwestern region of the major ocean. As
is the case of the ocean general circulation modeling, the
EKWC, a western boundary current in the East Sea, shows
a tendency to separate from the coast at higher latitudes than
at the observed ones in the previous numerical models, and
328
C.-H. Kim and J.-H. Yoon
2. Numerical Model
The numerical model used in this study is the Modular
Ocean Model (MOM, Pacanowski et al., 1991, 1993) version 1.1 which is the successor to the Cox version (1984).
This model assumes the Boussinesq approximation, hydrostatic balance and rigid-lid surface in the spherical coordinate. The model covers an area from 33°N to 52°N in
latitude and from 126°30′ E to 142°30′ E in longitude.
Horizontal grid resolution is 1/6 degree in both longitude
and latitude, so the zonal grid size varies from 15.5 km to
11.5 km to the north and the meridional grid size is constant
as 18.5 km. The model inlets are composed of two sections
(Fig. 1): the westernmost section from the south coast of
Korea to the Cheju Island, and the southernmost section
from the Cheju Island to the west coast of Japan (Kyushu).
A realistic bottom topography is introduced by taking up to
19 vertical levels in the deepest part using the ETOPO5
global depth data (NCAR, 1989). The vertical grid intervals
with tracer and velocity point at the center of the grid are,
from the top level, 15, 15, 20, 25, 30, 35, 35, 35, 50, 75, 100,
150, 200, 300, 450, 600, 600, 600, 600 in meter.
For the boundary condition of momentum at the sea
surface, the monthly mean wind stress computed by Na et al.
(1992) is used. For the heat flux at the sea surface the
monthly mean heat flux calculated from the climatological
data by Hirose et al. (1996) are specified as the Haney type
heat flux (Haney, 1971), which is expressed as
QT = Q1 + Q2(Ta – θ1),
where Q1 and Q2 are monthly heat flux components, Ta is air
temperature, and θ1 is potential temperature of the first level
in the model. For the salt flux, the Newtonian type restoring
boundary condition is applied using the observed sea surface
salinity. The restoring time scale is given as 10 days. At the
lateral wall the non-slip, insulating, and impermeable
boundary conditions are applied. The initial conditions for
the tracers (potential temperature and salinity) are given by
the observed mean values in January. Both for the initial
condition and the surface boundary conditions, long-term
monthly mean values of the data from Japan Oceanographic
Data Center (JODC), Korea Fisheries Research and Development Agency (KFRDA, 1986) and Far Eastern Hydrometeorological Institute of Russia (FEHMI) are used.
The coefficients of horizontal and vertical eddy viscosities are taken as 3.0 × 106 cm2 /s and 1.0 cm2/s, respectively. For the diffusion of the tracers the isopycnal mixing
scheme is adopted (Redi, 1982; Cox, 1987) instead of a
constant horizontal mixing. Considering the effective
isopycnal mixing in the upper layer, the isopycnal mixing
coefficient Khl is given as
stress, and heat flux used for the surface and inflow boundary conditions are interpolated into daily values in the model
integration. After about 15 years of model integration, the
upper ocean above 1000 m depth repeats almost the same
seasonal changes except for some small scale variabilities
related to the eddy activities in the surface layer. We carried
out the model run for 30 years. The results after 30 year run
are shown here.
Khl = Khl(–H) + (Khl(0) – Khl(–H))exp(–z/1000)
3.1 Comparison of the calculated tracer fields with the
observation
3.1.1 Temperature
Figure 2 shows the horizontal distributions of mean
temperature at 90 m depth in the model and the observed
temperature at 100 m depth in February (upper) and August
(lower), respectively (JODC, 1978). The observed temperatures are the mean values over a one-degree interval.
Therefore they show smoothed spatial patterns. The model
temperature distributions are similar to the observed patterns
in general. In some area, however, the model results show
stronger thermal fronts compared to the observed ones: the
Polar Front region in the central part of the ocean, the coastal
regions along the Korean coast and the Japanese coast in
summer. A tongue-like temperature pattern is found off the
Korean coast both in February and August in the observed
field (Fig. 2). It can be seen more clearly from the local
observations (KFRDA, 1986). This kind of pattern is reproduced in the model as shown by the warm water belt
along the Korean coast in August, indicating a northward
advection of the EKWC.
One of the major differences between the model and
the observed results is the latitude of the Polar Front and the
insufficient cooling in the model: the position of the Polar
Front in the model is shifted about 1° northward compared
to the climatological mean position. The temperature in the
cold water region north of the Polar Front is warmer than the
observed ones, especially in winter. For example, it is in the
range of 3~5°C in the most of the cold water region in
February, which is 3~4°C higher than the observation. The
temperature in the warm water region is also warmer than
the observed ones.
The cause for the insufficient cooling in the model
compared to the observed results seems to be related to the
accuracy of the heat flux data used. The heat flux was
calculated mainly from the COADS (Comprehensive OceanAtmosphere Data Set), which shows very small number of
observations in the northern part of the East Sea, especially
in the cold season (Hirose et al., 1996). Therefore, long-term
monthly mean values of heat flux were used in this model.
Incorporation of a high frequency component in the heat
flux exchange seems to enhance a cooling in the model
(Stanev, 1994).
after England (1993) and Hirst and Cai (1994). This indicates that the isopycnal mixing coefficient decreases from
Khl(0) at the surface level (5.0 × 105 cm2/s) to Khl (–H) at the
deepest level (1.0 × 105 cm2/s) in the model. To avoid the
numerical instability the background horizontal diffusivity
is set to 1.0 × 105 cm2/s and the maximum slope of the
isopycnal surface is limited to 1/50. The vertical diffusivity
coefficient (Kv) varying with depth has been tested by many
authors in order to see the effect of a larger vertical diffusion
in a deep layer (Weaver and Sarachik, 1990; Cummins et al.,
1990; England 1993; Hirst and Cai, 1994). We employ the
vertical diffusivity coefficient which is the minimum from
the surface to 385 m depth (0.1 cm2/s) and then increases
linearly to the bottom (0.4 cm2/s). In regions of the static
instability a convective adjustment process is solved implicitly with a larger vertical diffusivity of 104 cm2/s. In this model
deep convection does not reach to the bottom in winter. In
order to prevent the water from warming at a deeper depth
a robust-diagnostic term (Semtner and Chervin, 1988) is
inserted from the 16th (1835 m) to 19th (3635 m) level,
where the damping time scale of the predicted tracers to the
observed values is given as 3 years.
Many studies have been reported on the volume transport of the TC through the Korea/Tsushima Straits, but the
exact values are not yet known (for review, refer to Kim,
1996). In this model we assume a simple sinusoidal and
seasonal variation of the TC transport through the inlets with
the minimum in mid-March and the maximum in midSeptember. The annual mean of the inflow transport is taken
to be 2.2 Sv (1 Sv = 106 m3/s) and the amplitude of seasonal
variation 0.35 Sv based on the observation by Isobe (1994).
The volume transport through the Tsugaru Strait is fixed to
1.4 Sv without the seasonal variation considering the ADCP
observation by Shikama (1994), and the rest of the transport
occurs through the Soya Strait. The inflow and outflow
boundary conditions on the barotropic and baroclinic
components of velocities are the same as those used by Yoon
(1991a). The seasonally varying temperature and salinity
are imposed at each inflow boundary based on the observed
mean values by KFRDA (1986).
All the variables such as temperature, salinity, wind
3. Model Results
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
329
Fig. 2. Horizontal distributions of mean temperature in February and August (left; model, right; observation).
3.1.2 Salinity
Fresh waters with salinity less than 34.0 psu appear in
the model off the continental coast and Japanese coast in
February as shown in the observation (Fig. 3). The fresh
330
C.-H. Kim and J.-H. Yoon
water in the northwestern part of the model persists in
summer over a relatively large area. A saline water more
than 34.5 psu enters through the Korea/Tsushima Straits in
February and then decreases slowly to 34.1 psu toward the
Fig. 3. Horizontal distributions of mean salinity in February and August (left; model, right; observation).
central part of the model. Because of this inflow, salinity in
the interior is higher in August than in February. These
features in the model show a general agreement with the
observation. In August a saline water with salinity more than
34.3 psu is extended from the Korea/Tsushima Straits to the
Tsugaru Strait, flowing along the Japanese coast. In the
model, however, it remains in the central part.
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
331
Fig. 4. Monthly variations of horizontal velocity at 22.5 m depth in the model.
332
C.-H. Kim and J.-H. Yoon
Fig. 4. (continued).
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
333
Fig. 4. (continued).
334
C.-H. Kim and J.-H. Yoon
Fig. 5. Distributions of the horizontal velocity at 192.5 m depth in 15th of July and August in the model.
3.2 Seasonal variations of the Tsushima Current and the
Cold Currents
3.2.1 Current features in the Korea/Tsushima Straits
Figure 4 shows the monthly mean velocity field at 22.5
m depth (the second level) of the model. The TC is separated
into two streams around the Tsushima Island. The current
passing through the eastern channel continues flowing
northeastward along the Japanese coast and forms the
Nearshore Branch (NB). The current flowing through the
western channel diverges at the channel outlet. A part of the
current flows northward along the Korean coast and forms
the EKWC. The other part flows northeastward and joins the
NB. The TC shows a steady flow pattern in the Korea/
Tsushima Straits except for the strengthening of the current
in summer due to the increase of volume transport and
baroclinicity.
At the southwest of the Oki Bank (130°20′ E~131°30′
E, 35°10′ N~35°50′ N) where the depth deepens abruptly
from 200 m to more than 1000 m, a part of the flow turns
northwestward, and then joins the EKWC. The
northwestward turning of the flow as shown here occurs
year round, appearing more clearly from June to August.
3.2.2 The Nearshore Branch
In the distribution of the horizontal velocity at 22.5 m
depth (Fig. 4) the Tsushima Current flows over the shelf
region along the San’in coast up to 35°30′ N, forming the
NB. From January to May, the NB flows over the continental
shelf along the coast of Honshu up to the Noto Peninsula,
while a part of the flow detours to the north at the Oki Bank
along the shelf slope roughly following the 200 m isobath.
From June to July the NB becomes strong along the entire
continental slope extending to the Tsugaru Strait.
From August through September, small meanders begin to grow in some downstream areas passing the Oki Bank.
These meanders grow large in October. In November and
December the well-developed meanders start being isolated,
forming a separated eddy. The NB itself also departs from
the coast and moves offshore due to the β-effect. In winter
the NB becomes weak together with the decay of these
eddies.
One may notice that there is a countercurrent flowing
southwestward underneath the NB from south of 40°N to the
Oki Bank (Fig. 5). The subsurface countercurrent becomes
strongest from July to October. Figure 6(a) shows the zonal
component of velocity in 15th of June on the north-south
section along 135°E. The NB flows eastward with the
velocity of about 25 cm/s near the coast. Under the NB there
exists a countercurrent with the westward component of 5
cm/s. Hase et al. (1996) conducted ADCP and CTD measurements on the sections crossing the shelf slope between
135°E~137°E in May and June 1995 and found the southwestward countercurrent below the TC near the coast (Fig.
6(b)). In the observation the northeastward component of
the TC was more than 30 cm/s and the southwestward
component of the subsurface countercurrent was 5~10
cm/s at the depths of 150~300 m over the shelf region.
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
335
Fig. 6. Vertical distributions of (a) the zonal component of velocity in 15th June on the north-south section along 135°E in the model
and (b) the velocity component normal to the section from 36°33′ N, 134°49′ E (Sta. 1) to 35°50′ N, 135°18′ E (Sta. 13) observed
in May, 1995 (after Hase et al., 1996).
Fig. 7. Distributions of the horizontal velocity at 122.5 m depth in 15th of April and June in the model.
336
C.-H. Kim and J.-H. Yoon
Fig. 8. Distributions of the horizontal velocity at 385 m depth in 15th of February, March, April and May in the model.
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
337
338
C.-H. Kim and J.-H. Yoon
Fig. 9. Vertical distribution of salinity on the section E (shown
in Fig. 11) in December, 1987. Hatched area shows the
salinity minimum layer (after Kim, et al., 1991).
Fig. 10. Monthly variations of salinity on the section E (shown in Fig. 11) from February
to June with meridional component of velocity in February.
Fig. 10. (continued).
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
339
3.2.3 The East Korean Warm Current
The EKWC flows along the east coast of Korea and
then separates from the coast at about 37°N latitude at 22.5
m depth from January to August (Fig. 4). From summer to
autumn the northward flowing EKWC becomes strong. It
separates from the coast at about 39°N latitude in November.
There appears a southward countercurrent at 22.5 m
depth at the offshore side of the EKWC in January. This
southward countercurrent becomes strong from March
probably due to the strengthening of the EKWC. In April
and May it forms almost a closed anticyclonic recirculation
in the Ulleung Basin. The recirculation of the EKWC, which
was also simulated in Seung and Kim (1993), appears more
clearly at 122.5 m depth in the Ulleung Basin (Fig. 7). In
June it grows as large as the size of the Ulleung Basin. It lasts
throughout a year at 122.5 m depth, and also appears at 385
m depth from April to October (Fig. 8). Its vertical scale is
over 400 m.
Another small anticyclonic eddy starts growing at the
northern end of the EKWC around 38°N, 130°E from June
through July (Fig. 4). This eddy develops into an another
recirculation gyre in September at 38°N~39°30′ N,
129°E~131°E to the north of the recirculation gyre in the
Ulleung Basin. The northern recirculation becomes larger
than the southern one in October. It persists to the next
March and then breakes into small eddies before disappearing in May.
From the recent observations using CTD, ADCP and
satellite-tracked drifters an anticyclonic motion with a horizontal scale of 120~160 km and a vertical scale of about 300
m was observed in the Ulleung Warm Water region (Shin et
al., 1995; Lie et al., 1995). This eddy-like motion has persisted for several months. It is supposed that the clockwise
motion of the Ulleung Warm Water is strongly associated
with the EKWC recirculation. This is a part of the process to
diffuse the excessive negative vorticity in the western
boundary current region in the model.
There also appears a cyclonic eddy to the northeast of
the anticyclonic recirculation in the Ulleung Basin from July
to December (Fig. 4). This cyclonic eddy is a detached one
during the meandering process of the offshore extension of
the EKWC from March through July. However, its existence is not yet clearly supported by the observations.
3.2.4 The Liman Current/The North Korean Cold Current
The Liman Current (LC) originates in the northernmost
region of the East Sea (Fig. 4). It flows southwestward along
the Siberian coast and is extended by the North Korean Cold
Current (NKCC) south of 43°N. The LC is strengthened by
the winter monsoon and is the strongest from December to
March in the surface layer. The maximum speed is about 20
cm/s in January. It has a barotropic structure with a speed of
about 10 cm/s at 385 m depth (Fig. 8).
In the surface layer the southern limit of the NKCC is
restricted by the northward flowing EKWC, but in the
340
C.-H. Kim and J.-H. Yoon
subsurface layer it reaches more to the south: at 385 m depth
it extends almost to the Noto Peninsula in January and
February, flowing counterclockwise along the continental
slope. From April the southward flow of the NKCC is
hindered by the development of the EKWC and its
recirculation in the Ulleung Basin.
3.3 Circulation in the intermediate layer
3.3.1 Observation of the salinity minimum layer
The East Sea Intermediate Water (ESIW) found in the
southern part of the Ulleung Basin by Kim and Chung
(1984) is characterized by the salinity-minimum and dissolved oxygen-maximum in the subsurface layer. Cho and
Kim (1994) suggested two modes of the Salinity Minimum
Layer (SML) less than 34.00 psu in the Ulleung Basin: the
North Korean Cold Water (NKCW) and the ESIW. They
reported that the NKCW was observed only along the
eastern coast of Korea in summer, whereas the ESIW was
observed around the Ulleung Island in April, spreading
southward subsequently within the Ulleung Basin. The
depth of the SML was observed to be 100 m~200 m for the
NKCW and 200 m~400 m for the ESIW. According to Kim
et al. (1991) the low salinity core in the SML was observed
also in December near the Korean coast (Fig. 9).
3.3.2 Salinity minimum layer in the model
Figure 10 shows the vertical distribution of salinity and
the meridional component of velocity on the zonal section at
the latitude of 37°35′ N (the section E in Fig. 11). In February waters of high salinity with more than 34.10 psu originated from the TC water appear from the surface to about
300 m depth. Below this high salinity water the SML
represented by the isohaline of 34.04 psu is formed. The core
of the low salinity in the coastal region becomes fresher than
in January (not shown here), and a part of it surfaces near the
coast. This feature is very similar to that observed by Kim et
al. (1991) at the same section (Fig. 9). It is important to note
that the appearance of the low salinity core near the Korean
coast is related with the NKCC flowing southward in the
subsurface layer as indicated by the meridional component
of velocity. The NKCC is strongest in the surface layer near
the coast and shows significant speed down to 500 m depth.
In March the core of the SML near the coast of Korea
deepens and becomes more saline. It disappears in May.
Another isolated low salinity water less than 34.02 psu
appears at the depths of 400~500 m at 132°E~133°E in
March and is connected with the low salinity water in the
coastal side in April. In May the core of the salinity minimum with salinity less than 34.00 psu appears at 130°20′
E~130°40′ E west of the Ulleung Island and develops to a
larger one in June. Figure 11 shows the horizontal distributions of salinity at 385 m depth. Considering the salinity
field (Fig. 11) and the velocity field (Fig. 8) together, it can
be known that the low salinity water appeared in the offshore
region in March and April is the one which remained near
the Oki Bank for a longer time. This low salinity water is
Fig. 11. Horizontal distributions of salinity at 385 m depth in February, April and June in the model. The low salinity water between
33.98 and 34.00 psu is indicated with dot.
advected northward by the cold current flowing counterclockwise in the Ulleung Basin in March and April. However, the patch of the low salinity water appeared in May and
June is the one transported from the north to the Ulleung
Basin as an offshore continuation of the coastal core.
Figure 12 shows the distributions of temperature and
salinity on the meridional section along 130°E (the section
N in Fig. 11). In December there exists a fresh water with the
salinity of 33.80~33.95 psu from the surface to about 150 m
depth in the north of the Polar Front formed at about 40°N.
The low salinity water is mixed vertically by the winter
convection from January through March as indicated by the
surfacing of the 4~5°C isotherms and the steepening of the
isohalines of 33.92~33.98 psu.
In the horizontal distributions of salinity the low salinity water is found along the northeastern coastal region of
Korea and Siberian coast (Fig. 11). The dotted part shows
the region with the salinity between 33.98 and 34.00 psu
corresponding to the salinity range of the core water in the
SML. It exists most abundantly near the East Korean Bay
throughout a year. During winter season the well-mixed low
salinity water off the northeast coast of Korea is advected
southward along the coast of Korea at the depths of 100~500
m, refreshing the SML in the Ulleung Basin. In the simulation of drifter tracking using the velocity field in this model
the drifters released along 40°N in the EKB in the 1st of
January arrived at the section E within two months (Kim,
1996). Considering those results obtained in the drifter
experiments, it is most probable that the newly formed core
water of the salinity minimum layer in February on the
section E is originated in the EKB. From April the southward advection of the low salinity water near the latitude of
the E section shifts from the coastal side to the offshore
region due to the development of the EKWC along the
Korean coast. In the Ulleung Basin the spatial distribution of
the low salinity water is affected by the recirculating gyre of
the EKWC: the salinity-minimum core water rotates
clockwise with the EKWC recirculation from April to June.
In summer the supply of the southward flowing low salinity
water is diminished with the weakening of the NKCC, and
the salinity-minimum core water is diffused away in the
Ulleung Basin.
Deep currents were observed at the depths below the
SML off the mid-east coast of Korea from the end of August
to November 1986 (Lie et al., 1989). The deep currents
measured at 620 m and 790 m depth were directed to
southeast with an average speed of about 3 cm/s. In the
model the currents at 585 m and 785 m depth near the
location observed by Lie et al. (1989) are southeastward from
September to the next March and fluctuate with a period of
about 1 month in the rest of a year. The average speed is
about 0.7 cm/s from September to November and about 2
cm/s from December through March.
4. Discussion and Conclusion
Figure 13 shows the distributions of stream function.
The maximum volume transport in the northern cyclonic
gyre is up to 5 Sv in February. The separation of the EKWC
from the coast occurs at about 37°N in February and south
of 39°N for the rest of the year. This indicates that the
overshooting of the EKWC is properly suppressed compared to the previous experiments (Yoon, 1991a; Seung and
Kim, 1993).
In the reduced-gravity model experiment by Kim and
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
341
342
C.-H. Kim and J.-H. Yoon
Fig. 12. Vertical distributions of temperature and salinity on the section N (shown in Fig. 11) in 15th of December, January and February
in the model.
Fig. 13. Distributions of stream function in February, May, August and November in the model (unit in Sv, 1 Sv = 106 m3/s).
Yoon (1996), the wind stress with the positive curl in winter
season (Na et al., 1992) played an essential role in the
formation of the cyclonic gyre in the region north of the
Polar Front and the proper separation of the EKWC. How-
ever, incorporation of the density diffusion in the reducedgravity model resulted in a northward shift of the EKWC
separation latitude. This result suggests that a larger horizontal diffusion in the model dissipates the positive vorticity
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
343
of the northern recirculation gyre, and thus weakens the
southward flow of the NKCC, leaving the EKWC to overshoot.
In the present study we employed an isopycnal mixing
scheme for the diffusion of the tracers in which an isopycnal
mixing coefficient is coupled with a density gradient. Therefore, the horizontal diffusion of heat transported mainly by
the EKWC could be greatly suppressed across the thermal
front near the Korean coast. We also applied the realistic
buoyancy forcing (Hirose et al., 1996) with a relatively small
vertical diffusivity in the surface layer. These factors contribute to keep the northern waters cool and to maintain the
density structure of the cold water similar to the observed
one.
Kawabe (1982) and Yoon (1991b) pointed out that the
NB is topographically controlled near the Oki Bank. The
present model also shows a strong seasonal variation of the
NB near the Oki Bank: it flows over the shelf in spring (for
example, May as shown in Fig. 4), but in summer the
offshore current over the shelf slope region becomes stronger (June and July in Fig. 4). A part of the flow departs away
from the shelf slope due to the β-effect during summer and
autumn (September to November in Fig. 4).
East of the Oki Bank, branching of the TC into socalled the first and the second branches is not clear. Rather,
a single current system is flowing northeastward with the
development of a meander: the NB follows about 200 m
isobath from late winter to spring. During the period from
summer to autumn it meanders in the offshore region,
because of the relaxation from the topographic control. At
the fully developed meandering phase, eddies are generated.
The growth of meander in the NB appears to be due to
baroclinic instability. Ikeda and Emery (1984) and Ikeda et
al. (1984) discussed the meandering process caused by the
baroclinic instability in the California Current system with
some stability analyses and model experiments. As in the
California Current system, there exists a subsurface countercurrent flowing southwestward along the Japanese coast
under the northeastward surface current. Vertical shear in
the NB system and the coastal geometry like the shelf slope
are responsible for the growth of meander via the baroclinic
instability mechanism.
North of the Polar Front, especially in the northeastern
coastal region of Korea, the subsurface water is well mixed
with the surface fresh water due to the winter convection
which is reaching to about 500 m depth and leaves low
salinity less than 34.00 psu. The NKCC plays an important
role in transporting this low salinity water to the south along
the Korean coast. Cho and Kim (1994) reported the southward extension of the NKCW from June to August along the
coast of Korea. In the model the southward supply of the low
salinity water by the NKCC is stronger in winter and
becomes weak in summer, which is inconsistent with the
observation by Cho and Kim (1994). In the confluence zone
344
C.-H. Kim and J.-H. Yoon
between the NKCC and the EKWC, this cold and low
salinity water is advected to the depths of 200~500 m,
forming the SML beneath the warm and saline EKWC
water. Spatial distribution of the salinity minimum core in
the Ulleung Basin is associated with the development of the
EKWC and its recirculation gyre. When the EKWC
recirculation gyre strengthens in the Ulleung Basin, the
clockwise motion of the recirculation gyre directs the salinity-minimum core water offshore and spreads it further
south.
Recent findings on the circulation in the East Sea are
featured by eddies in both warm and cold water regions
(Kim et al., 1996), which are difficult to resolve in a linear
model with a coarse grid. In this study we tried to simulate
the upper and the intermediate layer circulation using a
multi-level numerical model with a relatively high resolution. Some of the simulated results successfully captured the
observed features of the TC and the SML, although those
observed features are also based on a very limited number of
observations. Therefore, the circulation shown in this numerical model should be verified by more detailed observations.
Acknowledgements
We express our hearty thanks to Prof. Takematsu of
Kyushu University for his encouragement during this research. We are grateful to the two anonymous reviewers for
their critical reading and useful comments. Thanks are also
due to Dr. J.-Y. Yun of KORDI who carefully read the
manuscript. The numerical calculation was carried out on a
FACOM VP2600 in the Computer Center at Kyushu University.
References
Bang, I., J.-K. Choi, L. Kantha, C. Horton, M. Clifford, M.-S. Suk,
K.-I. Chang, S. Y. Nam and H.-J. Lie (1996): A hindcast
experiment in the East Sea (Sea of Japan). La mer, 34, 108–
130.
Beardsley, R. C., R. Limeburner, K. Kim and J. Candela (1992):
Lagrangian flow observations in the East China, Yellow and
Japan Seas. La mer, 30, 297–314.
Bryan, F. and W. R. Holland (1989): A high resolution simulation
of the wind- and thermohaline-driven circulation of the North
Atlantic Ocean. p. 99–116. In Parameterization of Small Scale
Processes, ed. by P. Mueller, Hawaii Institute of Geophysics
Special Publication, University of Hawaii, Honolulu.
Cherniawsky, J. and G. Holloway (1993): On western boundary
current separation in an upper ocean general circulation model
of the North Pacific. J. Geophy. Res., 98, 22843–22853.
Cho, Y. K. and K. Kim (1994): Characteristics and origin of the
cold water in the south sea of Korea in Summer. J. Oceanol.
Soc. Korea, 29, 414–421.
Cox, M. D. (1984): A primitive equation, 3-dimensional model of
the ocean. GFDL Ocean Group Technical Report No. 1.,
GFDL/Princeton Univ.
Cox, M. D. (1987): Isopycnal diffusion in a z-coordinate ocean
model. Ocean Modelling, 74, 1–5.
Cummins, P. F., G. Holloway and A. E. Gargett (1990): Sensitivity
of the GFDL ocean general circulation model to a parameterization of vertical diffusion. J. Phys. Oceanogr., 20, 817–830.
England, M. H. (1993): Representing the global-scale water
masses in ocean general circulation models. J. Phys. Oceanogr.,
23, 1523–1552.
Ezer, T. and G. L. Mellor (1992): A numerical study of the
variability and the separation of the Gulf Stream, induced by
surface atmospheric forcing and lateral boundary flows. J. Phys.
Oceanogr., 22, 660–682.
Haney, R. L. (1971): Surface thermal boundary conditions for
ocean circulation models. J. Phys. Oceanogr., 1, 241–248.
Hase, H., J.-H. Yoon and M. Takematsu (1996): The measurement
of the southwestward subsurface countercurrent off the Wakasa
Bay. Proc. CREAMS’96, Vladivostok, 113–117.
Hirose, N., C.-H. Kim and J.-H. Yoon (1996): Heat budget in the
Japan Sea. J. Oceanogr., 52, 553–574.
Hirst, A. C. and W. Cai (1994): Sensitivity of a world ocean GCM
to changes in subsurface mixing parameterization. J. Phys.
Oceanogr., 24, 1256–1279.
Holloway, G., T. Sou and M. Eby (1995): Dynamics of circulation
of the Japan Sea. J. Mar. Res., 53, 539–569.
Ikeda, M. and W. J. Emery (1984): Satellite Observations and
modeling of meanders in the California Current System off
Oregon and northern California. J. Phys. Oceanogr., 14, 1434–
1450.
Ikeda, M., L. A. Mysak and W. J. Emery (1984): Observation and
modeling of satellite-sensed meanders and eddies off
Vancouver Island. J. Phys. Oceanogr., 14, 3–21.
Isobe, A. (1994): On the Tsushima Warm Current in the Tsushima
Strait. Kaiyo Monthly, 802–809.
Japan Oceanographic Data Center (1978): Marine Environment
Atlas, Northwestern Pacific Ocean I (seasonal and monthly).
Japan Hydrogr. Association.
Kawabe, M. (1982): Branching of the Tsushima Current in the
Japan Sea. Part II: Numerical experiment. J. Oceanogr. Soc.
Japan, 38, 183–192.
Kawai, H. (1974): Transition of current images in the Japan Sea.
p. 7–26. In The Tsushima Warm Current—Oceanic Structure
and Fishery, ed. by Fishery Soc. Japan, Koseisha Koseikaku,
Tokyo (in Japanese).
Kim, C.-H. (1996): A numerical experiment study on the circulation of the Japan Sea (East Sea). Doctoral Dissertation,
Kyushu Univ., 151 pp.
Kim, C.-H. and J.-H. Yoon (1996): Modeling of the wind-driven
circulation in the Japan Sea using a reduced-gravity model.
experiment. J. Oceanogr., 52, 359–373.
Kim, C.-H., H.-J. Lie and K.-S. Chu (1991): On the intermediate
water in the southwestern East Sea (Sea of Japan). p. 129–141.
In Oceanography of Asian Marginal Seas, ed. by K. Takano,
Elsevier, Amsterdam.
Kim, K. and J. Y. Chung (1984): On the salinity minimum layer
and dissolved oxygen-maximum layer in the East Sea (Japan
Sea). p. 55–65. In Ocean Hydrodynamics of the Japan and
East China Sea, ed. by T. Ichiye, Elsevier, Amsterdam.
Kim K., K.-R. Kim, Y.-G. Kim, Y.-K. Cho, J.-Y. Chung, B.-H.
Choi, S.-K. Byun, G.-H. Hong, M. Takematsu, J.-H. Yoon, Y.
Volkov and M. Danchenkov (1996): New findings from
CREAMS observations: water masses and eddies in the East
Sea. J. Korean Soc. Oceanogr., 31, 155–163.
Korea Fisheries Research and Development Agency (1986): Mean
Oceanographic Charts of the Adjacent Seas of Korea. Pusan,
Korea, 186 pp.
Lie, H.-J., M. S. Suk and C. H. Kim (1989): Observations of
southeastward deep currents off the east coast of Korea. J.
Oceanol. Soc. Korea, 24, 63–68.
Lie, H.-J., S.-K. Byun, I. Bang and C.-H. Cho (1995) Physical
structure of eddies in the southwestern East Sea. J. Oceanol.
Soc. Korea, 30, 170–183.
Na, J.-Y., J.-W. Seo and S.-K. Han (1992): Monthly-mean sea
surface winds over the adjacent seas of the Korean Peninsula.
J. Oceanol. Soc. Korea, 27, 1-10.
Naganuma, K. (1977): The oceanographic fluctuations in the
Japan Sea. Mar. Sci. (Kaiyo Kagaku), 9, 137–141 (in Japanese
with English abstract).
National Center for Atmospheric Research (1989): NCAR ASCII
Version of ETOPO5 earth surface elevation. Data Support
Section.
Pacanowski, R. C., K. Dixon and A. Rosati (1991, 1993): The
GFDL Modular Ocean Model Users Guide, version 1.0,1.1,
GFDL Ocean Group Tech. Report No. 2.
Redi, M. H. (1982): Oceanic isopycnal mixing by coordinate
rotation. J. Phys. Oceanogr., 12, 1154–1158.
Semtner, A. J. and R. M. Chervin (1988): A simulation of the
global ocean circulation with resolved eddies. J. Geophys. Res.,
93, 15502–15522.
Seung, Y. H. and K. Kim (1993): A numerical modeling of the
East Sea circulation. J. Oceanol. Soc. Korea, 28, 292–304.
Shikama, N. (1994): Variation of volume transport in the Tsugaru
Strait measured by bottom-mounted ADCP. Kaiyo Monthly,
815–818.
Shin, H.-R., S.-K. Byun and C. Kim (1995): The characteristics of
structure of warm eddy observed to the northwest of Ullungdo
in 1992. J. Oceanol. Soc. Korea, 30, 39–56 (in Korean with
English abstract).
Stanev, E. V. (1994): Assimilation of sea surface temperature data
in a numerical ocean circulation model. A study of the water
mass formation. p. 33–57. In Data Assimilation: Tools for
Modelling the Ocean in a Global Change Perspective, ed. by
P. P. Brasseur and J. C. J. Nihoul, NATO ASI Series, 19.
Takematsu, M., H. Hase, Z. Nagano and T. Kita (1996): Moored
instrument observations in the Japan Sea Proper Water. Proc.
CREAMS’96, Vladivostok, 101–105.
Weaver, A. J. and E. S. Sarachik (1990): On the importance of
vertical resolution in certain ocean general circulation models. J. Phys. Oceanogr., 20, 600–609.
Yoon, J.-H. (1982a): Numerical experiment on the circulation in
the Japan Sea, Part I. Formation of the East Korean Warm
Current. J. Oceanogr. Soc. Japan, 38, 43–51.
Yoon, J.-H. (1982b): Numerical experiment on the circulation in
the Japan Sea, Part III. Mechanism of the nearshore branch of
the Tsushima Current. J. Oceanogr. Soc. Japan, 38, 125–130.
Yoon, J.-H. (1991a): The seasonal variation of the East Korean
Warm Current. Rep. Res. Inst. Appl. Mech. Kyushu Univ., 38,
23–36.
Yoon, J.-H. (1991b): The branching of the Tsushima Current.
Rep. Res. Inst. Appl. Mech. Kyushu Univ., 38, 1–21.
A Numerical Modeling of the Upper and the Intermediate Layer Circulation in the East Sea
345