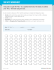
DMA 070 Worksheet #1 Simplifying, Multiplying, and

DMA 070 Worksheet #1
Simplifying, Multiplying, and Dividing Rational Expressions
Show your work. If the problem is a real world application or "word problem", write a complete sentence as your
final answer.
Find all numbers for which the rational expression is not defined.
2
1)
a-8
2)
3)
c- 8
6
x2 - 16
x2 - 2x - 24
Simplify.
4)
30m5p2
5m10p
5)
3m2 + 3m
6m2 + 21m
6)
4x + 4
12x2 + 20x + 8
7)
y 2 + 7y - 18
y 2 + 16y + 63
1
Multiply and, if possible, simplify.
2z3 ∙ 8
8)
4
z2
9)
2p - 2 ∙ 4p2
p
7p - 7
10)
k 2 + 12k + 32 ∙ k 2 + 6k
k 2 + 14k + 48 k 2 + 2k - 8
11)
x4 - 16 ∙ x2 + 1
x4 - 1 x2 + 4
Divide and simplify.
2x2 ÷ x3
12)
3
12
13)
7p - 7 ÷ 9p - 9
p
8p2
14)
x2 + 7x + 12 ÷ x2 - 9
x +4
4x - 12
15)
a2 - 8a + 7 ÷ a2 - 2a + 1
a2 + 14a + 49 a2 - 12a + 35
2
16) Evaluate the following rational expression when x = -3, x = 0, and x = 2.
2x3+7x-6
x2-4
17) The formula t= 40(25+1.85a) relates the time t, in minutes that it takes to cook an average-size potato in an
50-1.85a
oven that is at an altitude of a thousands of feet. Calculate the time it takes to cook a potato at an altitude of
3500. Then, find how long it would take to cook a potato at 7000 feet. Round all answers to the nearest
minute.
18) Based on the two answers calculated in the potato problem, can you make a conjecture about the length of
time it takes to cook an average-size potato in relation to the altitude?
19) In 1992, the U.S. Department of Transportation recommended that states adopt 0.08% blood-alcohol
concentration (BAC) as the legal measure of drunk driving. If you assume that a regular 12oz beer is 5%
alcohol by volume and the normal bloodstream contains 5L of fluid, your maximum BAC percentage can be
600n
approximately modeled by the rational function, B=
, where n is the number of beers consumed
w(169+0.6n)
in one hour and w is your body weight in pounds. What BAC would a 160 pound person register if he/she
consumed 4 beers in an hour? Round to the nearest hundreth of a percent. Hint: Your answer is already
given as a percent since B is the BAC percentage.
3
Answer Key
Testname: DMA 070 WORKSHEET 1
1) a = 8
2) None
3) x = 6 and x = -4
6p
4) 5
m
5)
m +1
2m + 7
6)
1
3x + 2
7)
y-2
y +7
8) 4z
8p
9)
7
10)
k
k-2
11)
(x + 2)(x - 2)
(x + 1)(x - 1)
12)
8
x
13)
56p
9
14) 4
15)
(a - 7)2(a - 5)
(a + 7)2(a - 1)
16) -81/5 when x =-3, 3/2 when x=0, undefined when x=2
17) 29 min when x=3.5 (3500 feet), 41 min when x=7 (7000 feet)
18) As the altitude increases, the amount of cooking time increases.
19) .09%
4
DMA 070 Worksheet #2
Adding and Subtracting Rational Expressions
Show your work. If the problem is a real world application or "word problem", write a complete sentence as your final
answer.
Find the LCM.
1) 50x5y , 30x4y 3 , 15x2y 4
2) t , t - 7
3) m2 - 2 m , m2 - 3 m + 2
4) x2 - 3 x + 2 , x2 + 4 x - 12
Add. Simplify, if possible.
9 + 4
5)
15x 15x
6)
m2 - 8m + 15
m-5
m-5
7)
4
+ 6
1 -m m- 1
8)
3
7
+
7x- 8
8 - 7x
9)
3 + 9
14x 10x2
1
10)
7 + 8
r
r -5
11)
2
+ 5
2
y - 3y + 2 y 2 - 1
12)
6 + 4
x3y xy 5
Subtract. Simplify, if possible.
17 - 10
13)
q-4 q-4
14)
3x + 26 x + 14
2
2
x + 4x - 12 x + 4x - 12
15)
9 - 4
9 -y y- 9
16)
3x - 24 - 3x - 24
x2 - 64 64 - x2
17)
3 - 9
10x 14x2
18)
x
x2 - 16
-
7
x2 + 5x + 4
2
19)
4x
x2 - 5x + 6
-
16
x2 - 6x + 8
Perform the indicated operation. Simplify, if possible.
-64 x +
1
- 3
20)
3 (8x + 1) 3 x( 8 x + 1)
x
21) Find the perimeter of the rectangle below. Explain a way that you can check your work.
3
Answer Key
Testname: DMA 070 WORKSHEET 2
1) 150 x5y 4
2) t(t - 7 )
3) m(m - 2 )(m - 1 )
4) (x - 1 )(x - 2 )(x + 6 )
13
5)
15x
6) m - 3
-2
7)
1 -m
8)
-4
7x- 8
9)
3(5x + 21)
70x2
10)
15 r - 35
r(r - 5 )
11)
7y- 8
(y - 1)(y + 1)(y - 2)
12)
4x2 + 6y 4
x3y 5
13)
7
q-4
14)
2
x- 2
15)
13
9 -y
16)
6
x +8
17)
3(7x - 15)
70x2
18)
x2 - 6 x + 28
(x - 4)(x + 4)(x + 1)
19)
4(x - 6)
(x - 3)(x - 4)
20) - 8(x + 1 )
3x
21)
4
DMA 070 Worksheet #3
Solving Rational Equations, Proportions, and Problem Solving
Show your work. If the problem is a real world application or "word problem", write a complete sentence as your final
answer.
Solve the equation.
5 = 2 +8
1)
x
x
2)
3 + 8 =1
x
9
3)
x
x
=2
5
9
4) x + -24 = -5
x
5)
x-4 = 4
5
x +2
6)
2 = 6
x+2 x- 7
7)
x -5 = 5
x- 5
x- 5
8)
3 =
y
y
3y- 6
1
9)
5
1 +
1
=
4
x- 4
4 x - 16
10)
2 - 4 =
4
y +4
y -4
2
y - 16
11)
3 = 3-x
6- x x-6
12)
y +1 y
= y-3
y + 6 y 2 - 36 y - 6
13)
x - 1 = x2 + 1
x + 1 x - 1 x2 - 1
Solve the problem.
14) Martha can rake the leaves in her yard in 3 hours. Her younger brother can do the job in 4 hours. How long
will it take them to do the job if they work together?
15) A man rode a bicycle for 12 miles and then hiked an additional 8 miles. The total time for the trip was
5 hours. If his rate when he was riding a bicycle was 10 miles per hour faster than his rate walking, what was
each rate?
Find the ratio. Simplify, if possible.
16) David's paycheck for a week at the video store was $112.86. If he worked 27 hours that week, what was his
pay rate?
2
Solve the problem.
17) A quality-control inspector examined 260 calculators and found 10 of them to be defective. At this rate, how
many defective calculators will there be in a batch of 17,160 calculators?
For the pair of similar triangles, find the length of the indicated side.
18)
In the outer triangle, the shortest side has length 9, and the middle side has length 12. In the inner triangle,
the shortest side has length 3, the middle side has length 4, and the longest side has length 5. Find the length,
x, of the longest side of the outer triangle.
3
Answer Key
Testname: DMA 070 WORKSHEET 3
1)
3
8
2) 27
45
3)
2
4) -8, 3
5) 28
13
6) 2
7) No solution
8) 3 , 6
9) 5
10) -14
11) No solution
4
12)
3
13) No solution
12
14)
hr
7
15) Bike: 12 mph
Hike: 2 mph
16) $4.18/hour
17) 660
18) x = 15
4
DMA 070 Worksheet #4
Variation, Problem Solving, and Graphing Rational Functions
Show your work. If the problem is a real world application or "word problem", write a complete sentence as your
final answer.
Given the values of x and y, find an equation of variation in which y varies directly as x.
1) y = 12, when x = 3
2) y = 37.8, when x = 10.8
Solve the problem.
3) The distance a vehicle can travel at a fixed speed varies directly as time. If a vehicle travels 1200 miles in 40
minutes, how far will it travel in 50 minutes?
4) The amount of money that Pablo makes in a given week varies directly as the number of hours he works that
week. In a week in which he works 34 hours, he makes $493. How much will he make in a week in which he
works 54 hours?
5) According to Ohm's law, the electric current I, in amperes, in a circuit varies directly as the voltage V. When 8
volts are applied, the current is 2 amperes. What is the current when 11 volts are applied?
Find an equation of variation in which y varies inversely as x and the following is true.
6) y = 31, when x = 5
7) y = 0.375, when x = 24
Solve the problem.
8) The volume V of a gas varies inversely as the pressure P on it. The volume of a gas is 400 cm3 under a
pressure of 40 kg/cm2. What is the volume of gas when the pressure is 1600 kg/cm2.
9) The speed of a vehicle is inversely proportional to the time it takes to travel a fixed distance. If a vehicle
travels a fixed distance at 25 miles per hour in 20 minutes, how fast must it travel to cover the same distance
in 10 minutes?
1
10) If x varies inversely as v, and x = 10 when v = 9, find x when v = 18.
Find an equation of variation in which the following are true.
11) y varies directly as the square of x, and y = 2.4 when x = 4.
12) y varies jointly as x and w and inversely as z, and y = 9 when x = 2, w = 3, and z = 30.
5
13) y varies jointly as x and z and inversely as the product of w and p, and y =
32
when x = 4, z = 8, w = 6, and
240
p = 8.
Solve.
14) The gravitational attraction A between two masses varies inversely as the square of the distance between
them. The force of attraction is 2.25 lb when the masses are 4 ft apart, what is the attraction when the masses
are 6 ft apart?
15) Wind resistance or atmospheric drag tends to slow down moving objects. Atmospheric drag varies jointly as
an object's surface area A and velocity v. If a car traveling at a speed of 60 mph with a surface area of 34 ft2
experiences a drag of 244.8 N (Newtons), how fast must a car with 48 ft2 of surface area travel in order to
experience a drag force of 368.64 N?
16) At a fixed temperature, the resistance R of a wire varies directly as the length l and inversely as the square of
its diameter d. If the resistance is 0.42 ohm when the diameter is 1 mm and the length is 210 cm, what is the
resistance when the diameter is 2 mm and the length is 820 cm?
Find the domain of the expression.
8
17)
p-3
2
18)
19)
5x - 6
(3x - 2)(x + 7)
x2 - 36
x2 - 11x + 28
Find any vertical asymptotes.
20) f(x) = x - 6
x2 - 4
21) f(x) = x - 6
x2 + 3x
Use the graph to answer the question.
22) Find the horizontal and vertical asymptotes of the rational function graphed below.
y
10
8
6
4
2
-10 -8
-6
-4
-2
-2
2
4
6
8
10 x
-4
-6
-8
-10
3
23) Find the horizontal and vertical asymptotes of the rational function graphed below.
y
6
4
2
-6
-4
-2
2
4
6
x
-2
-4
-6
Solve the problem.
24) In the following formula, f(x) is the minimum number of hours of studying required to attain a test score of
x: f(x) = 0.38x . How many hours of study are needed to score 85?
100.5 - x
25) A company that prints textbooks has a fixed monthly cost of $150,000. It costs $10 to produce each textbook.
answer the following questions.
a. Write the cost function, C(x), of producing x number of textbooks.
b. Write the average cost function C(x), of producing x textbooks.
c. Find and interpret C (1,000), C (10,000), C (100,000).
d. What is the horizontal asymptote for the graph of C(x)? Describe what this represents for the company.
4
Answer Key
Testname: DMA 070 WORKSHEET 4
1) y = 4x
2) y = 3.5x
3) 1500 miles
4) $783
5) 2.75 amperes
6) y = 155
x
7) y = 9
x
8) 10 cu. cm.
9) 50 miles per hour
10) x = 5
11) y = 0.15x2
12) y = 9xw
z
13) y = xz
5wp
14) 1 lb
15) 64 mph
16) 0.41 ohm
17) {p|p ≠ 3}
18) {x|x ≠ 2 , -7
3
19) {x|x ≠ 4 and x ≠ 7}
20) x = 2, x = -2
21) x = 0, x = -3
22) Horizontal: y = -2; vertical: x = -1
23) Horizontal: y = -1; vertical: x = ±4
24) 2.08 hr
25) a. C(x)=150,000+10x
b.
c. $160, $25, $11.50
d. y=10 The more textbooks that are produced, the closer the average cost per book approaches $10.
This is the least possible cost per textbook.
5
DMA 070 Review
Show your work. If the problem is a real world application or "word problem", write a complete sentence as your
final answer.
Find all numbers for which the rational expression is not defined.
x2 - 9
1)
x2 - 6x + 8
Solve the problem.
2) If the average cost per unit C(x) to produce x units of plywood is given by C(x) = 900 , what is the unit cost
x + 30
for 20 units?
Simplify.
3)
15x2 - 60x
12x2 - 48x
4)
2x + 2
10x2 + 14x + 4
5)
y 2 - 3y - 10
y 2 + 9y + 14
Multiply and, if possible, simplify.
2p - 2 ∙ 6p2
6)
p
9p - 9
7)
k 2 + 9k + 20 ∙ k 2 + 5k
k 2 + 10k + 25 k 2 - 2k - 24
1
Divide and simplify.
x2 - 25 ÷ 8x + 40
8)
x
x-5
9)
z2 + 11z + 30 ÷
z2 + 5z
z2 + 13z + 42 z2 + 10z + 21
Add. Simplify, if possible.
6 + 7
10)
r
r- 3
11)
2
+ 7
2
y - 3y + 2 y 2 - 1
Subtract. Simplify, if possible.
1 - 1
12)
x +1
x-1
13)
7x
28
2
2
x - 5x + 6 x - 6x + 8
Solve the equation.
4 = 2 +5
14)
x
x
2
15)
4 = 6
x- 8 x+ 1
16)
-5x = 7x + 2x- 1
3x + 3
6x+ 6
x+ 1
17)
-1 - 7 =
8
y +4
y -4
2
y - 16
18)
8 = x
x- 8 x- 8
Solve the problem.
19) If x varies inversely as v, and x = 24 when v = 5, find x when v = 15.
Solve.
20) The volume of wood in a tree varies jointly as the height of the tree and the square of the distance around the
tree trunk. If the volume of wood is 15.84 cubic feet when the height is 22 feet and the distance around the
trunk is 3 feet, what is the volume of wood obtained from a tree that is 29 feet tall having a measurement of
4 feet around the trunk?
Find the domain and list the vertical asymptotes.
2
21) f(x) = x + 14x + 48
x2 - 1
3
Solve.
22) The current in a stream moves at a rate of 4 mph. If a boat travels 82 miles downstream in the same time that
it takes to travel 41 miles upstream, what is the speed of the boat in still water?
23) Martha can rake the leaves in her yard in 2 hours. Her younger brother can do the job in 5 hours. How long
will it take them to do the job if they work together?
For the pair of similar triangles, find the length of the indicated side.
24)
In the upper triangle, the shortest side has length 22, and the middle side has length 33. In the lower triangle,
the shortest side has length 18, the middle side has length 27, and the longest side has length 36. Find the
length, x, of the longest side of the upper triangle.
Solve the problem.
25) A quality-control inspector examined 200 calculators and found 8 of them to be defective. At this rate, how
many defective calculators will there be in a batch of 15,800 calculators?
4
Answer Key
Testname: DMA 70 REVIEW
1) x = 4 and x = 2
2) $18.00
5
3)
4
4)
1
5x + 2
5)
y-5
y+7
6)
4p
3
7)
k
k-6
8)
(x - 5)2
8x
9)
z+3
z
10)
13 r - 18
r(r - 3 )
11)
9 y - 12
(y - 1)(y + 1)(y - 2)
12)
-2
2
x -1
13)
7(x - 6)
(x - 3)(x - 4)
14)
2
5
15) 26
6
16)
29
17) -4
18) No solution
19) x = 8
20) 37.12 cubic feet
21) (-∞, -1) ∪ (-1, 1) ∪ (1, ∞)
22) 12 mph
3
23) 1 hr
7
24) x = 44
25) 632
5