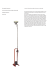Modelling of an infrared halogen lamp in a rapid thermal system

International Journal of Thermal Sciences 49 (2010) 1437e1445
Contents lists available at ScienceDirect
International Journal of Thermal Sciences
journal homepage: www.elsevier.com/locate/ijts
Modelling of an infrared halogen lamp in a rapid thermal system
P.O. Logerais a, *, A. Bouteville b
a
b
Université Paris-Est, Centre d’Études et de Recherche en Thermique Environnement et Systèmes (CERTES), IUT de Sénart, Avenue Pierre Point, 77127 Lieusaint, France
Arts et Métiers ParisTech, LAMPA, 2 boulevard du Ronceray, BP 93525, 49035 Angers Cedex 01, France
a r t i c l e i n f o
a b s t r a c t
Article history:
Received 24 November 2009
Received in revised form
26 February 2010
Accepted 8 March 2010
Available online 10 April 2010
The heat flux distribution of an infrared halogen lamp in a Rapid Thermal Processing (RTP) equipment is
studied. An overview of lamp modelling in RTP systems is given and for the first time, the infrared lamp
bank is modelled by taking into consideration with accuracy a lamp portion in the bank environment.
A three-dimensional (3D) lamp model, with a fine filament representation is largely presented. The
model assumptions are in particular exposed with focusing on the thermal boundary conditions. The
lamp temperature is calculated by solving the radiative heat transfer equation by means of the MonteCarlo method for ray tracing. Numerical calculations are performed with the finite volume method.
A very good agreement is found with experimental data in steady state. The heat amount provided by the
lamp is also determined. As a first development, transient calculations are performed with the validated
model and the dynamic behaviour of the lamp during heating process is determined with precision.
Lastly, the model is discussed and further developments are proposed.
Ó 2010 Elsevier Masson SAS. All rights reserved.
Keywords:
Infrared halogen lamp
Rapid Thermal Processing (RTP)
Modelling
Numerical simulation
Monte-Carlo method
Lamp temperature
1. Introduction
Rapid Thermal Processes (RTP) are essential in the
manufacturing of semiconductor devices such as integrated circuits,
memories or solar cells. They correspond to key stages in the wafer
production operations like annealing (RTA), oxidation (RTO) or
Chemical Vapour Deposition (RTCVD) [1e3]. As feature size
decreases towards the nanometre scale and wafer diameter
increases, a deep knowledge of the phenomena involved in the
processes is crucial. Indeed, there is a growing demand from rapid
thermal equipment manufacturers and users to improve control,
uniformity and repeatability of wafer processes. As wafer temperature requirement is especially moving to a drastic 1 K precision,
wafer heating has to be mastered with high accuracy. Development
of numerical tools has accompanied with success the evolution of
RTP over the last two decades. Numerical tools have allowed a better
understanding of the various aspects of the processes such as wafer
heating, gas flow, thin film deposition, system control etc. Heat and
mass transfer have been namely simulated by using the Computational Fluid Dynamics (CFD) method in an efficacious way [4,5].
In RTP systems, a silicon wafer is heated up at a very high rate by
the radiative heat provided by halogen infrared lamps (Fig. 1a).
Process times vary from a few seconds for implant annealing up to
* Corresponding author. Tel.: þ33 1 64 13 46 86; fax: þ33 1 64 13 45 01.
E-mail address: [email protected] (P.O. Logerais).
1290-0729/$ e see front matter Ó 2010 Elsevier Masson SAS. All rights reserved.
doi:10.1016/j.ijthermalsci.2010.03.003
a few minutes for high-K annealing or curing [6]. The main technological challenge is to obtain a well controlled uniform temperature at the wafer surface. So the perfect knowledge of radiative
heat emitted by the infrared lamps is necessary. The infrared lamps
are usually arranged in banks in the furnace of RTP equipments
(Fig. 1b). For information, in a cold wall reactor, the wafer is placed
in a chamber and the wall is kept cooled by means of a water flow.
A quartz window separates the chamber from the furnace. The
radiative heat is transported from the lamps to the wafer through
the quartz window and by reflections on the chamber wall.
A controller, commonly of Proportional Integral Derivate (PID) type,
connected to a pyrometer fixes the input lamp power to respect the
setpoint wafer temperature.
Halogen infrared lamps consist of a tungsten filament in
a middle of a quartz bulb (Fig. 1a). The latter is filled with nitrogen
under around 4 bar of over pressure to reduce the tungsten filament evaporation. Halogen gases with Iodine (I), Bromine (Br),
Chlorine (Cl) or Fluorine (F) are added. The created halogen cycle
helps tungsten redeposition on the filament. By this method, the
lamp lifetime and lamp brightness are increased. Then, the tungsten filament and the electrical power to apply can both remain
stable. The lamp bases containing the connectors must be kept
under 600 K. Consequently, pulsed air is flowed on the lamp bases
during process.
The RTP systems were modelled in different ways. The realized
models tend to be more and more accurate to best follow the trends
of microelectronic manufacturing requirements.
1438
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
Nomenclature
Ai
Al
Aop
Aop,l
Ast
Ast,l
cp
d
e
h0
i
I(r,U)
Ib(r)
k
kl
L
M
Mij
!
n
nl
~l
n
Ns
p
p0
P
Patm
qi
Qi
r
Rop
Rop,l
Rst
Rst,l
Sh
!
SM
t
T
area of the patch i surface
spectral absorptivity
absorptivity for an opaque surface
spectral absorptivity for an opaque surface
absorptivity for a semi-transparent parallel geometry
volume
spectral absorptivity for a semi-transparent parallel
geometry volume
specific heat capacity
radiation travelled distance
distance between the centres of two neighbour lamps
specific total enthalpy
specific internal energy function of the temperature T
and the density r
radiation intensity function of both position
r and direction U
intensity of blackbody radiation at the temperature of
the medium
thermal conductivity
spectral absorption index
length of the lamp
molar mass
radiation exchange matrix (fraction of radiation
emitted by patch i and absorbed by patch j)
unit normal vector at the surface location
spectral refractive index
spectral complex refractive index
total number of patches
static pressure
initial pressure
lamp applied power
atmospheric pressure
heat flux density for the patch i
heat flux of the patch i
radial position
reflectivity for an opaque surface
spectral reflectivity for an opaque surface
reflectivity for a semi-transparent parallel geometry
volume
spectral reflectivity for a semi-transparent parallel
geometry volume
additional source term namely the one due to
radiative transfer
additional momentum source term
time
temperature
Plévert et al. represented the lamp banks of an RTP equipment as
a continuous surface emitting infrared radiation [7]. This assumption led to an overestimation of the radiation emitted since only the
lamp filaments radiate.
Balakrishnan and Edgar used two ways for modelling lamps in
the RTP equipment they considered [8]. Firstly, the relationship
between the lamp power and the wafer temperature is evaluated
from the heat balance of the whole system. This relationship is valid
for lamps in steady state because temperature variations are low.
Secondly, the wafer temperature response and the lamp power
regulation system are identified as transfer functions. The
dynamics of the lamps are returned by a first order model. The time
constant of the lamp depends on the filament temperature Tfil. It is
proportional to T3
fil .
Tfil
Tj
Tst
Tst,l
T0
u, v, w
!
V
x, y, z
filament temperature
average temperature of patch j
transmissivity for a semi-transparent parallel
geometry volume
spectral transmissivity for a semi-transparent parallel
geometry volume
initial temperature
velocity components in the x, y and z directions
velocity vector
Cartesian coordinates
Greek symbols
al
spectral absorption coefficient
al,q
spectral and directional absorptivity
dij
Kronecker delta
D4long longitudinal net exchanged heat flux between two
adjacent portions
D41e2
lateral net exchanged heat flux between lamp 1 and
lamp 2
k
absorption coefficient
3
surface emissivity
3j
emissivity of the patch j
3l
spectral emissivity
3l,q
spectral and directional emissivity
l
radiation wavelength
n
kinematic viscosity
U
propagation direction of the outgoing radiation beam
U0
propagation direction of the incoming radiation
beam
4bot
average heat flux at the bottom of the furnace portion
41/2
heat flux emitted by lamp 1 towards lamp 2
F(U)
phase function of the energy transfer from the
incoming direction to the outgoing direction U
r
density
r
surface reflectivity
s
scattering coefficient
s
StefaneBoltzmann constant (5.669 108 W m2 K4)
s
transmissivity
sij
viscous stress tensor
q
radiation direction
q1
angle of incidence with the surface normal in the
medium 1
q2
angle of refraction with the surface normal in the
medium 2
Subscripts
i
patch number
j
patch number
Kersch and Schafbauer modelled a rapid thermal system in
which measured values for the lamp power are entered in the
studied RTP system model [9].
Habuka et al. studied an RTP system with circular lamps [10]. The
filament lamp is modelled by source points. The DARTS method
(Direct Approach using Ray Tracing Simulation) is used for ray
tracing. The lamp connectors are taken into consideration in the
model equations and their effect is found significant on the wafer
temperature which is lower by a few percent just below them.
Chao et al. calculated view factors and approximated the radiative properties [11]. The representation of the lamps in the model
is a set of concentric rings. A uniform applied power is treated.
Lamps are represented in the same way in other modelling works
like the one of Park and Jung [12].
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
1439
Fig. 1. a) Infrared halogen lamp. b) Rapid Thermal Processing (RTP) system configuration.
Chang and Hwang modelled linear infrared lamps by representing the complete lamp geometry [13]. The boundary conditions
are applied to the quartz bulb and to the filament: their absorptivities, their transmissivities and the applied heat power are
entered. Ray tracing and simulation of the absorption, reflection,
refraction and diffusion of the light are performed by using the
TracePro and ANSYS programs as well as the Monte-Carlo method.
A 1 kW lamp power is supplied.
To continue all these efforts, in a previous work, we (Logerais
et al.) modelled an RTP equipment [14]. As the lamp filament
consists of a large number of turns which are very close one to the
other, its representation in three dimensions has been approximated by a hollow cylinder with an imposed uniform temperature
in its outer surface. However, this representation involves a greater
tungsten volume than the one of the real helix shaped filament. So,
for a given supplied electrical power, the hollow cylinder representation should lead to an overestimated filament temperature.
In the present work, our aim is to determine the heat distribution of an infrared halogen lamp in an RTP system according to the
supplied electrical power. In the model, a high accurate representation of the filament is realized. The model is presented with
emphasis on the equations and on the assumptions. Numerical
calculations are performed to achieve better knowledge of the
thermal behaviour during thermal processes. In a last part, the
realized lamp model and its results are discussed.
2. Lamp model
In this work, the infrared lamp used in the AS-One 150 equipment developed by the AnnealSys Company is modelled [15]. The
lamp shape and arrangement of this RTP system are common to
most of the RTP systems, thus the presented results can be transferred to a lot of other systems.
The portion is in fact a slice of the furnace taken at mid-distance
between a lamp and its direct neighbour lamps respectively to the
right and to the left. Its width is therefore equal to the distance
between the centres of two adjacent lamps. Its length is equal to
one tenth of the lamp. Finally, its height is equal to the one of the
upper part of the furnace. This representation can be first justified
by the results obtained in Caratini’s work [16]. The latter indicates
that the heat flux emitted by the filament remains almost uniform
along it. Even in the presence of support rings and also close to the
edges of the lamp, the emitted flux decrease is low (maximum of
5%). So considering one tenth of the lamp is representative. The
portion dimensions will be more justified in the below part 2.2 and
2.5 with the mesh cell number and the boundary conditions.
2.2. Meshing
The realized meshing for the lamp is shown on Fig. 2. The lamp
filament was achieved by generating helix curves with CFD’GEOM
software [17]. The number of whorls and the distance between two
consecutive turns were specified after being measured with
a calliper rule. A hybrid mesh was entered. The filament and
nitrogen trapped in the bulb quartz is a tetragonal unstructured
mesh whereas the quartz bulb and the air in the portion are represented by a structured mesh. The size of the cells was chosen to
be as most balanced as possible to facilitate the numerical solving.
The domain is composed of around 150 000 cells. This huge cell
number ensures a very accurate representation of the filament, so
the view factors are implicitly known perfectly. Furthermore, this
model is less time consuming because a full filament lamp representation would involve an enormous number of cells and nonending calculations.
2.1. Geometry
2.3. Equations
The model consists of a three-dimensional (3D) representation
of one tenth of the lamp in a furnace portion (Figs. 2 and 3a).
The equations governing the conservation of mass, momentum
and energy are solved [18]. The mass conservation equation and the
1440
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
Fig. 2. Lamp model geometry with its mesh.
momentum conservation equation are respectively given by
expressions (1) and (2).
!
vr
þ div r V ¼ 0
vt
(1)
!
!
v rV
! !
! !
þ r V div V ¼ gradp þ mD V þ S M
vt
(2)
!
!
vðrh0 Þ
vp
vðusxx Þ v usyx
þ divðr V h0 Þ ¼ divðkgradTÞ þ
þ
þ
vt
vt
vx
vy
v vsxy
v vsyy
vðuszx Þ
þ
þ
þ
vz
vx
vy
v vszy
vðwsxz Þ v wsyz
þ
þ
þ
vx
vz
vy
vðwszz Þ
þ Sh
ð3Þ
þ
vz
in which h0 is the specific total enthalpy defined by the following
expression (4):
p
r
þ
1 2
u þ v2 þ w2
2
(4)
!
One can remark that vp/vt, divð V sij Þ and (1/2) (u2 þ v2 þ w2) are
negligible in equations (3) and (4), and that the convective heat
transfer can be overlooked with respect to the radiative heat
transfer. So, afterwards, the flow field is not shown.
The radiative heat transfer equation for an emitting-absorbing
and scattering medium can be presented as found below [18,19].
!
UgradðIðr; UÞÞ ¼ ðk þ sÞIðr; UÞ þ kIb ðrÞ
Z
0
0
s
Iðr; UÞF U /U dU
þ
4p
(5)
0
U ¼ 4p
!
where, UgradðIðr; UÞÞ is the gradient of the intensity in the specified
propagation direction U; (k þ s)I(r,U) represents the changes in
intensity due to absorption k and out-scattering s; kIb(r)
R
U0 ¼ 4p
Iðr; UÞFðU0 /UÞdU0 is the
gain due to in-scattering where F(U0 /U) is the phase function of
the energy transfer from the incoming U0 direction to the outgoing
U direction; and the intensity at the surface is evaluated by:
Iðr; UÞ ¼ 3Ib ðrÞ þ
r
p
Z
nU
The heat transfer is calculated by solving the energy conservation equation (3):
h0 ¼ i þ
corresponds to the emission; ðs=4pÞ
0 0
0
jnU jI r; U dU
(6)
0
The radiative heat transfer equation (5) is solved by using the
Monte-Carlo method. The used scheme is detailed in the work of
Mazumder and Kersch [20]. In this scheme, the rays emitted by each
surface of the system, called “patch”, are traced until they are
absorbed by the same surface or any other surface. Thus, the
equation (5) solution corresponds to a radiation energy exchange
between a given patch i and all the other patches j:
Qi ¼ qi Ai ¼
NS X
Mij dij 3j sTj4 Aj
(7)
j¼1
where NS is the total number of patches and Mij is the radiation
exchange matrix (fraction of radiation emitted by patch i and
absorbed by patch j).
A photon issued of a patch i, undergoes many events before
being absorbed by a surface. When radiation strikes a body, the
processes of absorption, reflection (diffuse, specular or partially
specular) and transmission can occur [21]. Each of these events will
depend on the radiation wavelength, the radiation propagation
direction, the patch orientation and the patch optical properties.
The optical properties are described by the complex refractive
~ l given by expression (8) which depends on the incident
index n
radiation wavelength and also the patch temperature [22]. It allows
to determine the absorptivity, emissivity, reflectivity and transmissivity of the different surfaces which can be semi-transparent or
opaque.
~ l ¼ nl ikl
n
(8)
For semi-transparent parallel geometry like the quartz bulb,
several assumptions presented in the book of Morokoff and Kersch
are considered like a constant absorption coefficient al in the
material volume [23]. The Snell law, the Fresnel formulas and
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
s ¼ edal
al ¼
4pkl
l
1441
(13)
(14)
in which q1 is the angle of incidence with the surface normal in the
medium 1, q2 is the angle of refraction with the surface normal in
the medium 2 and d is the radiation travelled distance.
For opaque surfaces, like the tungsten filament, obviously
transmissivity is equal to zero. The reflectivity is calculated
knowing the absorptivity:
Rop ¼ 1 Aop
(15)
In both the semi-transparent and opaque cases, the absorptivity
is equal to the emissivity for a spectral and a directional condition
as stipulated by Kirchhoff’s law [25]:
al;q ¼ 3l;q
(16)
Al ¼ 3l
(17)
Most of all, the Monte-Carlo method is used to determine the
wavelength, the direction and the trajectory of the photons from
their emission point to their absorption point. These characteristics
are obtained by inversing cumulated distribution functions [19].
Representative bundles of photons are considered. Their trajectories must be perfectly randomised to reproduce namely the diffuse
emission phenomena. Hence, the choice of the Monte-Carlo
method is fully justified.
2.4. Volume properties and initial values
Each part of the lamp (the tungsten filament, the nitrogen gas in
the bulb, the quartz bulb and the air in the furnace) is considered as
a volume. All the volume and initial data are reported in Table 1.
They come from different sources [26e28]. Viscosities, specific heat
capacities and thermal conductivities all vary with temperature.
The hypothesis of ideal gas law is supposed for the bulb nitrogen
and the air. The halogen gases in the bulb, which are in minor
quantities compared to nitrogen, are not introduced in the model.
2.5. Boundary conditions
Fig. 3. Choice of the dimensions and boundary conditions.
a matrix approach are used [24]. Absorptivity, reflectivity and
transmissivity for the semi-transparent parallel geometry are given
by the below expressions (9)e(11):
Ast ¼
ð1 rÞð1 sÞ
1 rs
"
Rst
ð1 rÞ2 s2
¼ r 1þ
1 r 2 s2
Tst ¼
(9)
#
ð1 rÞ2 s
1 r2 s2
(10)
(11)
where
r¼
!
1 tan2 ðq1 q2 Þ sin2 ðq1 q2 Þ
þ
2 tan2 ðq1 þ q2 Þ sin2 ðq1 þ q2 Þ
(12)
The boundary condition description will allow us to explain fully
the choice of the lamp representation. The dimensions and
boundary conditions of the portion are chosen to stay the closest to
the furnace conditions. The thermal conditions, in particular the
radiative boundary conditions of the portion are depicted on the
schematic diagrams of Fig. 3. The choice of the portion dimensions
is linked to the heat fluxes. The absorptivity, emissivity, reflectivity
and transmissivity for all the surfaces are shown on Fig. 4. Their
values are calculated according to the material optical data
provided by the book of Palik [22].
In Fig. 3b, a few lamps close one to the other in the furnace are
considered. This diagram is practical to show the influence between
lamps next to one another. In fact, in a lamp bank, the lamps are
mutually heated. This mutual heating occurs mainly between
a lamp and its first neighbour lamp (lamp number 1 and 2). In
a section located midway between the two adjacent lamps 1 and 2,
the heat flux emitted by the lamp 1 towards lamp 2 and the one
received by lamp 1 from the nearby lamp 2, noted respectively
41/2 and 42/1, are equal in absolute value and opposed regarding
the midway plan. The net exchanged flux D41e2 is therefore equal
to zero in this plan. Hence, an adiabatic boundary condition is
chosen for the lateral boundary condition of the portion.
1442
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
Table 1
Volume and initial properties.
Volume designation
Volume property
Value
Filament (tungsten)
Density, r
Initial temperature, T0
Specific heat capacity, cp
Thermal conductivity, k
19 300 kg m3
300 K
0.0255T þ 124.35 J K1 kg1
3.3 105T2 0.1144T þ 199.7 W m1 K1
Nitrogen in the bulb
Molar mass, M
Initial pressure, p0
Initial temperature, T0
Kinematic viscosity, n
Specific heat capacity, cp
Thermal conductivity, k
28 g mol1
400 000 Pa
300 K
7 106 þ 4 108T 6 1012T2 m2 s1
993.1 þ 0.161T J K1 kg1
0.0068 þ 7 105T 7 109T2 W m1 K1
Bulb (quartz)
Density, r
Initial temperature, T0
Specific heat capacity, cp
Thermal conductivity, k
2649 kg m3
300 K
212.3 þ 4.75T 6.26 103T2 þ 3.66 106T3 7.8 1010T4 J K1 kg1
0.96 þ 2.43 103T 2.29 106T2 þ 7.94 1010T3 W m1 K1
Air in the furnace
Molar mass, M
Initial pressure, p0
Initial temperature, T0
Kinematic viscosity, n
Specific heat capacity, cp
Thermal conductivity, k
29 g mol1
100 000 Pa
300 K
8 106 þ 4 108T m2 s1
951.71 þ 0.194T J K1 kg1
0.0078 þ 6 108T W m1 K1
An adiabatic boundary condition is also considered for the walls
in the longitudinal direction. When one moves along the axis of the
lamp, the heat flux is uniform. By considering two portions put one
beside the other, in the section between the two portions, the
emitted and received fluxes will cancel out (D4long ¼ 0). Hence, the
choice of an adiabatic condition is considered for the longitudinal
boundary condition of the portion.
The “mirror” radiative property, that is to say a perfectly
reflective surface, is chosen for the lateral and longitudinal walls.
Indeed, the rays emitted respectively by the nearby lamp and the
portion beside are equivalent to those reflected by these perfectly
reflective walls.
The upper wall which is the furnace wall is made of stainless
steel and its temperature is estimated at 573 K.
Finally, the convective and the radiative heat has to be evacuated, otherwise the temperature inside the portion is going to
increase indefinitely. The hot air evacuation outside the portion
towards the furnace is reproduced by applying an atmospheric
pressure outlet condition at 300 K at the bottom portion. This
boundary condition is called “hot air evacuation” on Fig. 3b and c.
To take into account the radiation evacuation out of the portion to
the rest of the thermal system, the bottom portion optical property
is considered like the one of a blackbody at 300 K with an
absorptivity equal to 1 (Fig. 4). This boundary condition is
mentioned on Fig. 3b by the name “far”. In an RTP system, there are
other radiations besides the ones emitted by the infrared lamps
centred on 1 mm. These radiations are for example emitted by the
silicon wafer or by the quartz window. Consequently, a “farfield”
condition is considered to take into account these incoming radiations with their various widespread wavelengths.
We can specify that the model is valid for lamps near the walls of
the furnace as well. The distance between the lamp and the furnace
wall is of the same order than the one between two adjacent lamps.
As the wall is very reflective, the boundary condition with the
mirror radiative property is then a good approximation.
2.6. Numerical calculations
The numerical calculations are performed using the finite
volume method which consists in integrating the conservation
equations (1)e(3) and (5) over each domain cell of the geometry
system [29,30]. A second order method is chosen for the spatial
resolution. The ray tracing is performed for five millions rays in
order to ensure an accurate result. An at least four order magnitude
decrease of the residual sum is made sure during calculations to
have a satisfactory convergence. The numerical calculations are
carried out by using the CFD’Ace code on a PC type AMD Athlon 64,
processor 32 with 1 Gb RAM. As an idea, the convergence is achieved for steady-state calculations in about 3 h and in about 12 h for
the transient state ones.
In a previous work, the lamp filament temperature had been
determined experimentally for five constant heating powers [14].
The filament resistance had been deduced from measurements of
the tungsten filament electrical voltage and intensity during heating processes. As the filament resistivity is function of temperature,
the filament temperature could be determined. Five percentages
had been studied: 10, 15, 20, 25 and 30% of the maximum lamp
power had been applied. The obtained filament temperatures were
within 1700 and 2300 K, which are commonly utilized values in
RTP processes. The values of the measured electrical power density
are entered in the present model for the tungsten filament in order
to compare the lamp temperatures. Firstly, steady-state calculations are performed and confronted to the above mentioned
experimental results. Secondly, transient simulations are performed with the unconditionally stable Euler first order method for
time solving.
3. Results and discussion
Fig. 5 shows the result of a steady-state calculation for a 25%
applied power. We can see the incandescent tungsten filament and
the hot gases and bulb around it. For each applied power, the filament temperature simulated varies by about 30 K around the
average filament temperature which represents a deviation less
than 2%. The lamp average temperature is then presented in all this
work.
Since variation of the filament temperature is very rapid in
transient state, the time response uncertainty is important. Thus,
a steady-state comparison is more reliable. The steady-state
calculated filament temperatures are confronted to the experimental ones on Fig. 6 versus the five lamp power values [14]. The
experimental uncertainty intervals are also indicated. A very good
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
1443
Fig. 4. Surface radiative properties: a) Tungsten of the filament. b) Quartz of the bulb. c) Steel of the upper wall. d) Mirror. e) Far of the hot air evacuation.
agreement can be noticed between experimental and calculated
filament temperatures. All the calculated values are within the
accuracy interval and the differences found are less than 5%. So, this
validated model allows an accurate estimation of the heat flux
density flowing towards the bottom of the furnace and the reaction
chamber. As this heat is evacuated out of the considered lamp
portion, its value is negative. This heat flux density is called 4bot. Its
absolute average value, j4botj is reported on Fig. 7 versus the lamp
applied power. The relation deduced from this figure will be
practical for the user to predict the heat flux received by the
substrate according to the applied power to the infrared lamps.
Moreover, this result is of great interest if we intend to study an
entire RTP system in which the lamp bank is difficult to represent
due to the numerous details. In fact, the upper part of the furnace
which includes the lamp bank can be simplified by a wall with an
imposed heat flux with the determined values of Fig. 7. This
simplification was realized in the work of Plévert et al. but the wall
heat flux values were just estimated [7].
This validated model can also be simulated in transient state.
Fig. 8 shows the simulated dynamics of the lamp for the five
considered constant heating power. It allows to evaluate the lamp
response time.
Thereafter, a reflection is done on the possible developments
that can be put forward to the present precise lamp model.
As a first remark, the modelling method exposed in the present
work is not limited to the AS-One 150 system. It can be applied to
model cylindrical halogen infrared lamps of other RTP equipments
by adapting the dimensions and the mesh. An application of this
method to spherical bulbs is possible too with a preliminary study
on the mesh to realize. Besides, the present model is interesting to
complete rapid thermal system models where the lamp temperature was entered as a source term, like the ones we realized or
those in other achievements [10,14]. The source term values can be
previously calculated with the lamp model before simulating the
system. Moreover, different power applied instructions can be
entered according to the processes [6,31]. The wafer temperature
1444
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
Fig. 5. The heated infrared lamp filament (25% applied power).
evolution can be related to the one of the lamps in order to optimise
the thermal budget received by the wafer. Furthermore, modelling
several nearby lamps would give even more precise results for the
filament temperature. A better knowledge of the mutual heating
would be provided, namely if the amount of electrical power is
different from one lamp to another. In the present study, a uniform
heating of all the lamps was taken into consideration. But, in many
rapid thermal systems, the lamps can be piloted by groups in order
to get a uniform wafer temperature [32,33].
Developments of the present model to study the internal
phenomena involved during RTP utilizations are also possible. With
increasing computer capacities, it would be interesting to model at
least one quarter of the lamp with its base to see the temperature
decrease towards the edge. Here, a uniform temperature was
considered and the portion is supposed to be taken more towards
the mid part. We can mention that the Joule effect in the filament
can be simulated by coupling the electrical current continuity
equation to the heat conservation equation in the model [18]. Going
further, by adding the rings in the model, their thermal influence on
both the filament and the wafer could be appreciated. In spite of the
high pressure and the halogen gases in the bulb, there is an inevitable reduction of the tungsten filament volume by evaporation
with use and ageing. So, the introduction of a kinetic model for the
tungsten evaporation would permit to find a relationship between
the lamp lifetime and the number of rapid thermal cycles.
As a final note, this model can be instrumental in controlling and
regulating the wafer temperature in a tight way during lamp heating
process. For example, it can be integrated as a predictive model. The
addition of a physical predictive model to the controller of RTP
machine permits an optimization of the thermal budget received by
the silicon wafer from the infrared lamps [8,34]. Specially, a wafer
temperature very consistent with the required one can be obtained
with a gradual rise before reaching the setpoint wafer temperature.
Fig. 7. Absolute average heat flux density calculated at the bottom of the portion, j4botj
versus the supplied power in steady state.
Fig. 8. Evolution of the simulated filament temperature for different lamp supplied
power.
Fig. 6. Comparison between the experimental filament temperatures and the calculated ones versus the supplied power in steady state.
P.O. Logerais, A. Bouteville / International Journal of Thermal Sciences 49 (2010) 1437e1445
Nevertheless, further developments are necessary for a wafer mass
production. The physical model must be as close as possible to reality
to master the wafer process control, what we endeavoured to with
the realisation of an accurate lamp model.
4. Conclusion
The infrared halogen lamp model developed in the present
study gives a better knowledge of the provided heat amount
according to the supplied power in the considered Rapid Thermal
Processing (RTP) system. In the present work, an overview of
infrared lamp models in RTP systems is first given. A new threedimensional deep model with a faithful representation of the lamp
filament with its helix shape is afterwards largely developed. The
model assumptions are indeed described with interest to the
surface radiative properties and the solving of the radiative heat
transfer equation by means of the Monte-Carlo method. The model
is validated in steady state by a very good matching between
experimental and simulated infrared lamp filament temperature. A
relation is established allowing the user to predict the heat flux
received by the substrate according to the applied power of the
lamps. This heat flux can be used to simplify forthcoming entire RTP
system models. Moreover, the dynamics of the lamp is better
understood by the calculated temperature responses realized.
Subsequently, they will allow to optimise the wafer thermal
budget. Many other developments are exposed in the last part in
order to go further in the mastering of lamp modelling accuracy, to
improve RTP system models and wafer control temperature.
Acknowledgments
The authors gratefully thank the French Ministry of Education
and Research and the AnnealSys Company, especially Mr. Franck
Laporte for his help and fruitful discussions and Mr. Éric Dupuy for
technical assistance.
References
[1] B. Liu, J. Gao, K.M. Wu, C. Liu, Solid State Communications 149 (17e18) (2009)
715e717.
[2] R.A. Ismail, D.N. Raouf, D.F. Raouf, Journal of Optoelectronics and Advanced
Materials 8 (4) (2006) 1443e1446.
[3] W.B. Dubbeldaya, K.L. Kavanagh, Journal of Crystal Growth 222 (1e2) (2001)
20e28.
[4] J.D. Chung, Y.M. Cho, J.S. Lee, C.H. Jung, Y.J. Choi, K. Jung, IEEE International
Symposium on Industrial Electronics 2 (2001) 1208e1212.
1445
[5] M.G. Giridharan, S. Lowry, A. Krishnan, American Society of Mechanical
Engineers Paper (1995) 11.
[6] J. Niess, S. Paul, S. Buschbaum, P. Schmid, W. Lerch, Materials Science and
Engineering B 114e115 (2004) 141e150.
[7] L. Plévert, S. Mottet, M. Bonnel, N. Duhamel, R. Gy, L. Haji, B. Loisel, Japanese
Journal of Applied Physics 34 (2A) (1995) 419e424.
[8] K.S. Balakrishnan, T.F. Edgar, Thin Solid Films 365 (2000) 322e333.
[9] A. Kersch, T. Schafbauer, Thin Solid Films 365 (2) (2000) 307e321.
[10] H. Habuka, M. Shimada, K. Okuyama, Journal of the Electrochemical Society
147 (12) (2000) 4660e4664.
[11] C.K. Chao, S.Y. Hung, C.C. Yu, Journal of Manufacturing Science and Engineering 125 (2003) 504e511.
[12] H.M. Park, W.S. Jung, International Journal of Heat and Mass Transfer 44
(2001) 2053e2065.
[13] P.C. Chang, S.J. Hwang, International Journal of Heat and Mass Transfer 49
(2006) 3846e3854.
[14] P.O. Logerais, D. Chapron, J. Garnier, A. Bouteville, Microelectronic Engineering
85 (2008) 2282e2289.
[15] AS-One 150 is an AnnealSys product, Montpellier, France. www.annealsys.com.
[16] Y. Caratini, Développement d’un four de recuit rapide, application à la croissance et à la nitruration de couches minces d’oxydes sur silicium, Ph.D. thesis,
Institut National Polytechnique, Grenoble, France, 1988.
[17] CFD Research Corporation, CFD’GEOM User Manual (2007) Huntsville: http://
www.esi-group.com/.
[18] CFD Research Corporation, CFD’Ace (U) Module Manual (2007) Huntsville:
http://www.esi-group.com/.
[19] M.F. Modest, Radiative Heat Transfer. McGraw-Hill International Editions,
1993.
[20] S. Mazumder, A. Kersch, Numerical Heat Transfer, Part B: Fundamentals 37
(2000) 185e199.
[21] R. Siegel, J.R. Howell, Thermal Radiation Heat Transfer. Taylor and Francis,
Washington DC, 1992.
[22] E.D. Palik, Handbook of Optical Constants of Solids. Academic Press, New York,
1998.
[23] A. Kersch, W.J. Morokoff, Transport Simulation in Microelectronics. Birkhäuser, Basel, 1995.
[24] R.M.A. Azzam, N.M. Bashara, Ellipsometry and Polarized Light. North-Holland,
Amsterdam, 1977.
[25] F. Incropera, D.P. Dewitt, Fundamentals of Heat and Mass Transfer. John Wiley
and Sons, New York, 1996.
[26] Data available from CEA/NASA: http://cea.grc.nasa.gov/index.html.
[27] Data available from NIST Webbook: http://webbook.nist.gov/chemistry/fluid/.
[28] R.B. Sosman, The Properties of Silica: an Introduction to the Properties of
Substances in the Solid Non-conducting State. Chemical Catalog Co., New
York, 1927.
[29] S.V. Patankar, Numerical Heat Transfer and Fluid Flow. Hemisphere Publishing
Corporation, McGraw-Hill Book Company, New York, 1980.
[30] H.V. Versteeg, W. Malalasekera, An Introduction to Computational Fluid
Dynamics, the Finite Volume Method. Longman, London, 1995.
[31] V.E. Borisenko, P.J. Hesketh, Rapid Thermal Processing of Semiconductors.
Plenum Press, New York, 1997.
[32] N. Acharya, V. Kirtikar, S. Shooshtarian, H. Doan, P.J. Timans, K.S. Balakrishnan,
K.L. Knutson, IEEE Transactions on Semiconductor Manufacturing 14 (3)
(2001) 218e226.
[33] A.J. Silva Neto, M.J. Fordham, W.J. Kiether, F.Y. Sorrell, Revista Brasileira De
Ciencias Mecanicas, Journal of the Brazilian Society of Mechanical Sciences 20
(1998) 532e541.
[34] S.J. Kim, Y.M. Cho, Control Engineering Practice 10 (11) (2002) 1199e1210.