Final Exam Solution Key
Transcription
Final Exam Solution Key
PHYSICS 311: Classical Mechanics – 2015 Final Exam Solution Key Solution Key 1. [25 points] Short Answer (5 points each) (a) A cyclist of mass, m has a quadratic drag coefficient of c. She is on level ground, and is assumed to be moving at a constant speed. i. How much power (energy/time) must she exert in order to maintain a constant speed? ii. Supposing that she can crouch down such that her cross-section (and thus, her drag coefficient decreases by a factor of 2, how much faster or slower will she be able to go (assuming that her power output remains constant)? Sol. i. The drag force is: F = cv 2 and thus the power needed is: P = cv 3 ii. If c0 = 1/2c, then v 0 = 21/3 v will yield the same power output. For reference, this is a boost of about 26%. (b) What are Kepler’s 3 laws of planetary motion? Sol. While in an ideal world, I’d want you to know these in order, there is no penalty for swapping them around. i. Planets trace out elliptical orbits with the sun at a focus. ii. The area traced out is equal in equal time. Conservation of angular momentum. iii. P 2 = a3 . (c) A particle moves in the x-y plane under the potential: κ U (x, y) = − 2 x + y2 What is the force on the particle? Sol. This is identical to the problem on the midterm, but in Cartesian coordinates. The Force is: F~ = −∇U = − 2κy 2κx ˆ ˆj = − 2κ rˆ i− 2 (x2 + y 2 )2 (x + y 2 )2 r3 1 (d) The potential in the previous problem is invariant under the transformation: x → x + y y → y − x where is a small number. Given the Lagrangian: L= 1 m(x˙ 2 + y˙ 2 ) − U (x, y) 2 what is the conserved quantity from Noether’s theorem? Sol. Knowing: ∂L =0 ∂ we get: d X ∂L ∂qi =0 dt ∂ q˙i ∂ This yields: d (mxy ˙ − myx) ˙ =0 dt This is simply angular momentum in the z-direction! (e) The Lagrangian of a charged particle in a magnetic field is: L= h i 1 ˙2 ~ r) , m~r − q V (~r) − ~r˙ · A(~ 2 ~ is the vector potential. where A In cartesian coordinates, what is the canonical momentum in the x-direction? Sol. It’s simply: ∂L px = = mx˙ + qAx ∂ x˙ 2 2. [20 points] An Atwood machine (pictured) connects two masses, m1 and m2 , one of which is on an inclined plane. They are looped over a pulley with an outer radius, R and a moment of inertia, I. Please, for the love of all that is good and physics-y, think carefully about the kinetic energy of the pulley. (a) Write down the Lagrangian of the system in terms of y (the height of m1 off the ground), x (the distance m2 moves down the plane), and φ (the rotation angle of the pulley), and their time derivatives. In this problem and in the next, you are free to set your zero points of potential energy however you like. Sol. It’s fairly straightforward (except for the potential energy of m2 ): L= 1 1 1 m1 y˙ 2 + I φ˙ 2 + m2 x˙ 2 − m1 gy + m2 gx sin θ 2 2 2 (b) The three coordinates above are coupled. Write down y and φ in terms of x, and y˙ and φ˙ in terms of x. ˙ Sol. The linear coordinates are related trivially: y = x ; y˙ = x˙ But, we have: φ=− x x˙ ; φ˙ = − R R The sign is not particularly important. (c) Write the Euler-Lagrange equation for x. Sol. 3 First, we need to start with the Lagrangian. We get: 1 I L= m1 + m2 + 2 x˙ 2 − (m1 − m2 sin θ)gx 2 R So: I m1 + m2 + 2 R x ¨ = −(m1 − m2 sin θ)g (d) Suppose the masses are selected so that the force you just derived exactly balance. First, what is the necessary relationship to balance them? Second, describe the subsequent motion if they start at rest and you give m1 a little push down the plane? Be quantitative. Sol. They balance, naturally, for: m1 = m2 sin θ If you give one side a push, there will still be no net force, so the mass will continue down the plane at a constant speed until it hits the ground. 4 3. [15 points] Consider a charged particle in an electric field. The Lagrangian can be written: 1 L = mx˙ 2 + QE0 x 2 where Q is the charge of the particle, and E0 is the strength of the electric field. (a) What is the Hamiltonian of the system? You’ll only get full credit if you compute the Hamiltonian using the general formalism. Also, be sure to express your answer in terms of the position and the canonical momentum. Sol. First, note: px = mx˙ so: H = px x˙ − L = 1 mx˙ 2 − QE0 x 2 or H= p2 − QE0 x 2m (b) Write down Hamilton’s equations for the system. Sol. ∂H = −QE0 = −p˙ ∂x ∂H p = = x˙ ∂p m As expected. (c) The particle starts at x = 0 at t = 0. Sketch the subsequent path of the particle through phase space. Sol. We can solve this explicitly: p = QE0 t and x= 1 QE0 2 t 2 m so x ∝ p2 So the phase space path is: 5 6 4. [25 points] A mass, m is attached to a central pivot point by a spring of spring constant, k, and unstretched length r0 . It is free to fly around the equatorial (r − φ) plane. There is no friction or gravity. (a) Write down the Lagrangian of the system. Sol. The Lagrangian is: L= 1 1 m(r˙ 2 + r2 φ˙ 2 ) − k(r − r0 )2 2 2 (b) Write the Euler Lagrange equations for this system. Sol. The Lagrangian quickly yields the Euler-Lagrange equations: m¨ r = mrφ˙ 2 − k(r − r0 ) and, for φ d 2 ˙ mr φ = 0 dt (c) Are there any conserved momenta for this system? If so, label them for future use. Sol. Yes. l = mr2 φ˙ (d) Write down the equation of motion using only r and r¨ (use conserved ˙ quantities to eliminate φ). Sol. 7 We’ve done this a number of times before. Noting: φ˙ = l mr2 we get an equation of motion: m¨ r= l2 − k(r − r0 ) mr3 (e) Given the constraints of the system (the conserved quantity specified in part c) write down an algebraic equation for the radius of a circular orbit. Note: You don’t actually need to solve the equation. It’s quite ugly. Sol. We’re solving for r¨ = 0. So: l2 = k(r − r0 ) mr3 (f) E.C. Under the assumption that the displacement from r0 is small, approximate the radius of the circular orbit. You may find the following relation useful: 1 1 ∆ ' 1 − 3 (r0 + ∆)3 r03 r0 Sol. This yields: l2 mr03 1−3 ∆ r0 − k∆ = 0 or ∆= 8 l2 /mr03 k + 3l2 /mr02 5. [15 points] A very small, light projectile of mass, m, is fired at a larger target, a sphere of mass, M m, and radius, R. For a solid sphere, the differential cross section is: 1 dσ = R2 dΩ 4 But for this problem, a scatter only “counts” if the projectile bounces backwards (that is, if the x-component of the velocity is negative). (a) What is the maximum impact parameter that will produce a backscatter? What is the corresponding back-scatter cross-section? There are several valid approaches here: You may use trig or you can infer from the differential cross section. Sol. Approach # 1: We can do this geometrically, as we did in class: We see that if there’s an impact parameter, b, then the angle with respect to the origin will be: sin α = b R The angle scattered is θ = 2α (as we did in class and in your book), so to back-scatter, θ = π/2. Thus, α = π/4. Recalling: √ 2 sin π/4 = 2 we get: √ 2 bm = R 2 so πR2 σ = πb2m = 2 9 Approach # 2: We can also reverse it and recognize that since the differential cross section is constant, the fraction of πR2 that will back-scatter is 1/2πR2 (giving the cross section above). From that, it’s easy to get the maximum cross section. (b) Suppose now, we have a field of these spheres, of density, n. How far can we expect a projectile to penetrate into the field before it bounces back? Please express your answer in terms of R, n, and, if relevant, the speed of the projectile. Sol. Even if you screwed up and put, for instance, σ = πR2 , we’ll get the right dimensionality. That is: 1 2 = nσ nπR2 λ= (c) We assumed that the masses of the targets were arbitrarily greater than the mass of the projectiles. What if that isn’t so? Suppose that: M = 3m and the projectile files in at speed, v0 . What is the relative velocity of the lab frame with respect to the center of mass frame? Sol. First off, in the center of mass frame, the targets will move backwards (just to get the sign right). The CM velocity is: vCM = 10 mv0 v0 = 4m 4
Similar documents
IIT JEE-Advanced 2012 Solved papers by Triumph Academy
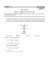 IIT JEE-Advanced 2012 Solved papers by Triumph Academy
IIT JEE-Advanced 2012 Solved papers by Triumph Academy










 -Triumph-Academy-JEE-Main
-Triumph-Academy-JEE-Main

 JEE Main 2015 Solved paper
JEE Main 2015 Solved paper

 Triumph-Academy JEE Main 2015
Triumph-Academy JEE Main 2015

