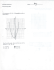Pre-Calculus 11 Quadratic Functions Worksheet.

Pre-Calculus 11
Quadratic
Functions Worksheet.
Pre-Calculus 11
Quadratic
Functions Worksheet.
1) Sketch each graph and find the y-intercept algebraically:
2) Write the equation of the parabola shown:
3) Write the equation for a parabola that:
a) opens up, has been stretched vertically by a factor of 2 and has been shifted 2
units right and 3 units down.
b) opens down, has been compressed vertically by a factor of
1
and has been
3
shifted 5 units left and 2 units up.
4) Write an equation for the parabola with the given information:
a) vertex (2, 5), congruent to , opening down y = 5 x 2
b) vertex (–4, 0), and passing through (–2, 12)
c) vertex (1, 3) and passing through the point (5, 7)
d) vertex (–2, –8) and a y–intercept of 4
e) congruent to y = 5(x + 7)2+1 with an axis of symmetry x = 3 and a max value of 5
5) Without graphing, state: i) the vertex, ii) equation of the axis of symmetry,
iii) domain and range, iv) y-intercept
v) min / max value and where it occurs
6) Sketch the graph of each parabola and label: i) the coordinates of the vertex
ii) the equation of the axis of symmetry,
iii) the max / min value and where it occurs
7) A farmer has 300m to enclose his livestock as shown.
What will the maximum area be?
P = 300 = 3 W + 2 L ; 2 L = 300 – 3 W
L = 150 – 1.5 W
A = LW = (150 – 1.5 W)W
= 150 W– 1.5 W2 = – 1.5 W2 + 150 W
Now, we have derived a quadratic equation to maximize the area:
Look for the vertex: (p, q) ;
p = – 150/2(– 1.5) = 50
q = – 1.5 (50)2 + 150 (50) = 3750
The maximum area is 3750 m2 when the W = 50 m and L = 75 m.
8) A theatre company sells tickets for $120 to 100 people. They estimate that for
every $5 decrease in price, 10 more people will purchase tickets. What price will
yield the maximum revenue?
Let x be the number of times that $ 5 decreases.
Revenue = ( 100 + 10x)(120 – 5x) = 12000 + 700x – 50x2
= – 50x2 + 700x + 12000
Again, we have derived a quadratic equation to maximize the revenue:
Look for the vertex: (p, q) ;
p = – 700/2(– 50) = 7
q = – 50 (7)2 + 700 (7) + 12000 = 14450
It seems that we need to decrease the price of $5 seven times:
$120 – $5 (7) = $ 120 – $ 35 = $ 85.
The maximum revenue will be $14450 if the new price is dropped to $85.